Girish Mahajan (Editor)

Gompertz distribution

Updated on
Edit
Like
Comment
Share on FacebookTweet on TwitterShare on LinkedInShare on Reddit
Gompertz distribution

Parameters
  
η , b > 0 {\displaystyle \eta ,b>0\,\!}

Support
  
x ∈ [ 0 , ∞ ) {\displaystyle x\in [0,\infty )\!}

PDF
  
b η e b x e η exp ⁡ ( − η e b x ) {\displaystyle b\eta e^{bx}e^{\eta }\exp \left(-\eta e^{bx}\right)}

CDF
  
1 − exp ⁡ ( − η ( e b x − 1 ) ) {\displaystyle 1-\exp \left(-\eta \left(e^{bx}-1\right)\right)}

Mean
  
( 1 / b ) e η Ei ( − η ) {\displaystyle (1/b)e^{\eta }{\text{Ei}}\left(-\eta \right)} where Ei ( z ) = ∫ − z ∞ ( e − v / v ) d v {\displaystyle {\text{where Ei}}\left(z\right)=\int \limits _{-z}^{\infty }\left(e^{-v}/v\right)dv}

Median
  
( 1 / b ) ln ⁡ [ ( − 1 / η ) ln ⁡ ( 1 / 2 ) + 1 ] {\displaystyle \left(1/b\right)\ln \left[\left(-1/\eta \right)\ln \left(1/2\right)+1\right]}

In probability and statistics, the Gompertz distribution is a continuous probability distribution, named after Benjamin Gompertz (1779 - 1865). The Gompertz distribution is often applied to describe the distribution of adult lifespans by demographers and actuaries. Related fields of science such as biology and gerontology also considered the Gompertz distribution for the analysis of survival. More recently, computer scientists have also started to model the failure rates of computer codes by the Gompertz distribution. In Marketing Science, it has been used as an individual-level simulation for customer lifetime value modeling. In network theory, particularly the Erdős–Rényi model, the walk length of a random self-avoiding walk (SAW) is distributed according to the Gompertz distribution.

Contents

Probability density function

The probability density function of the Gompertz distribution is:

f ( x ; η , b ) = b η e η e b x exp ⁡ ( − η e b x ) for  x ≥ 0 ,

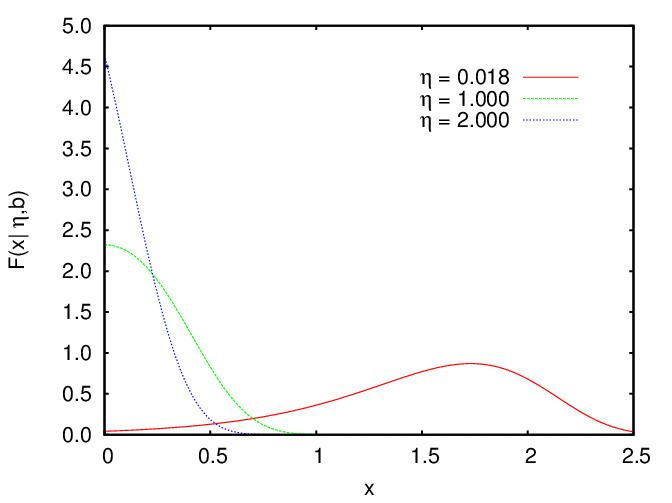
where b > 0 is the scale parameter and η > 0 is the shape parameter of the Gompertz distribution. In the actuarial and biological sciences and in demography, the Gompertz distribution is parametrized slightly differently (Gompertz–Makeham law of mortality).

Cumulative distribution function

The cumulative distribution function of the Gompertz distribution is:

F ( x ; η , b ) = 1 − exp ⁡ ( − η ( e b x − 1 ) ) ,

where η , b > 0 , and x ≥ 0 .

Moment generating function

The moment generating function is:

E ( e − t X ) = η e η E t / b ( η )

where

E t / b ( η ) = ∫ 1 ∞ e − η v v − t / b d v ,   t > 0.

Properties

The Gompertz distribution is a flexible distribution that can be skewed to the right and to the left. Its hazard function is a convex function of F ( x ; η , b ) . The model can be fitted into the innovation-imitation paradigm with p = η b as the coefficient of innovation and b as the coefficient of imitation. When t becomes large, z ( t ) approaches ∞ . The model can also belong to the propensity-to-adopt paradigm with η as the propensity to adopt and b as the overall appeal of the new offering.

Shapes

The Gompertz density function can take on different shapes depending on the values of the shape parameter η :

  • When η ≥ 1 , the probability density function has its mode at 0.
  • When 0 < η < 1 , the probability density function has its mode at

    • Kullback-Leibler divergence

    If f 1 and f 2 are the probability density functions of two Gompertz distributions, then their Kullback-Leibler divergence is given by

    D K L ( f 1 ∥ f 2 ) = ∫ 0 ∞ f 1 ( x ; b 1 , η 1 ) ln ⁡ f 1 ( x ; b 1 , η 1 ) f 2 ( x ; b 2 , η 2 ) d x = ln ⁡ e η 1 b 1 η 1 e η 2 b 2 η 2 + e η 1 [ ( b 2 b 1 − 1 ) Ei ⁡ ( − η 1 ) + η 2 η 1 b 2 b 1 Γ ( b 2 b 1 + 1 , η 1 ) ] − ( η 1 + 1 )

    where Ei ⁡ ( ⋅ ) denotes the exponential integral and Γ ( ⋅ , ⋅ ) is the upper incomplete gamma function.

  • If X is defined to be the result of sampling from a Gumbel distribution until a negative value Y is produced, and setting X=−Y, then X has a Gompertz distribution.
  • The gamma distribution is a natural conjugate prior to a Gompertz likelihood with known scale parameter b .
  • When η varies according to a gamma distribution with shape parameter α and scale parameter β (mean = α / β ), the distribution of x is Gamma/Gompertz.

    • References

    Gompertz distribution Wikipedia
    (Text) CC BY-SA