Multiple time dimensions

Physics

Special relativity describes spacetime as a manifold whose metric tensor has a negative eigenvalue. This corresponds to the existence of a "time-like" direction. A metric with multiple negative eigenvalues would correspondingly imply several timelike directions, i.e. multiple time dimensions, but there is no consensus regarding the relationship of these extra "times" to time as conventionally understood.

If thе special theory of relativity can be generalized for the case of k-dimensional time (t₁, t₂, ..., t_k) and n-dimensional space (x_k+1, x_k+2, ..., x_k+n), then the (k+n)-dimensional interval, being invariant, is given by the expression

(ds_k,n)² = (cdt₁)² + ... + (cdt_k)² − (dx_k+1)² − … − (dx_k+n)².

The metric signature will be

( + , ⋯ , + ⏟ k , − , ⋯ , − ⏟ n ) (timelike sign convention)

or

( − , ⋯ , − ⏟ k , + , ⋯ , + ⏟ n ) (spacelike sign convention).

The transformations between the two inertial frames of reference K and K′, which are in a standard configuration (i.e., transformations without translations and/or rotations of the space axis in the hyperplane of space and/or rotations of the time axis in the hyperplane of time), are given as follows:

t σ ′ = ∑ θ = 1 k ( δ σ θ t θ + c 2 v σ v θ β 2 ( ζ − 1 ) t θ ) − 1 v σ β 2 ζ x k + 1 , x k + 1 ′ = − c 2 β 2 ζ ∑ θ = 1 k t θ v θ + ζ x k + 1 , x λ ′ = x λ ,

where v 1 = ( v 1 , 0 , ⋯ , 0 ⏟ n − 1 ) , v 2 = ( v 2 , 0 , ⋯ , 0 ⏟ n − 1 ) , v k = ( v k , 0 , ⋯ , 0 ⏟ n − 1 ) are the vectors of the velocities of K′ against K, defined accordingly in relation to the time dimensions t₁, t₂, ..., t_k; β = 1 ∑ μ = 1 k c 2 v μ 2 ; ζ = 1 1 − β 2 ; σ = 1, 2, ..., k; λ = k+2, k+3, ..., k+n. Here δ_σθ is the Kronecker delta. These transformations are generalization of the Lorentz boost in a fixed space direction (x_k+1) in the field of the multidimensional time and multidimensional space.

Let us denote d x η d t σ = V σ η and d x η ′ d t σ ′ = V σ η ′ , where σ = 1, 2, ..., k; η = k+1, k+2, ..., k+n. The velocity-addition formula is then given by

V σ ( k + 1 ) ′ = V σ ( k + 1 ) ζ ( 1 − β 2 ∑ θ = 1 k c 2 v θ V θ ( k + 1 ) ) 1 + V σ ( k + 1 ) v σ β 2 ( ( ζ − 1 ) ∑ θ = 1 k c 2 v θ V θ ( k + 1 ) − ζ ) , V σ λ ′ = V σ λ 1 + V σ ( k + 1 ) v σ β 2 ( ( ζ − 1 ) ∑ θ = 1 k c 2 v θ V θ ( k + 1 ) − ζ ) ,

where σ = 1, 2, ..., k; λ = k+2, k+3, ..., k+n.

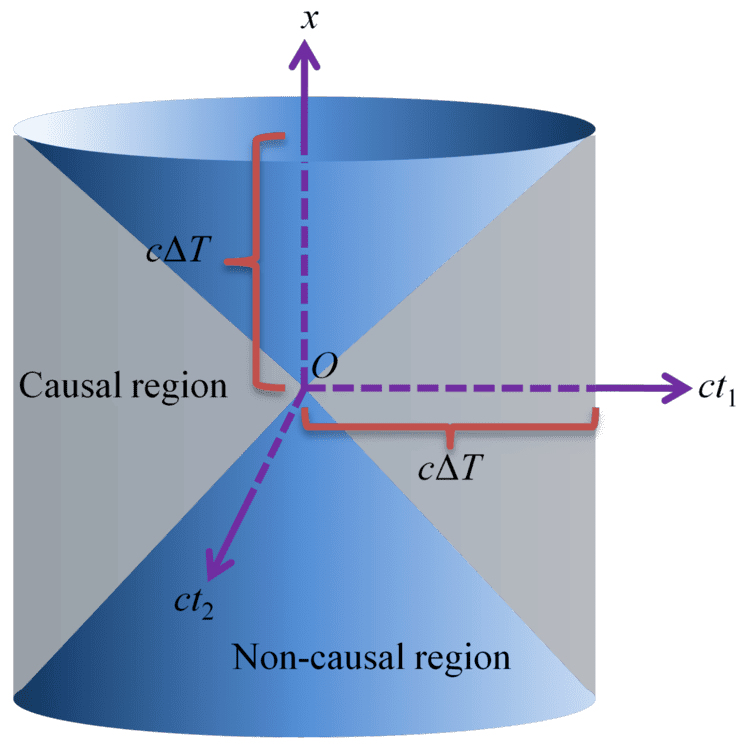
For simplicity, let us consider only one spatial dimension x₃ and the two time dimensions x₁ and x₂. (E. g., x₁ = ct₁, x₂ = ct₂, x₃ = x.) Let us assume that in point O, having coordinates x₁ = 0, x₂ = 0, x₃ = 0, there has been an event E. Let us further assume that a given interval of time Δ T = ( Δ t 1 ) 2 + ( Δ t 2 ) 2 ≥ 0 has passed since the event E. The causal region connected to the event E includes the lateral surface of the right circular cone {(x₁)² + (x₂)² − (x₃)² = 0}, the lateral surface of the right circular cylinder {(x₁)² + (x₂)² = c²ΔT²} and the inner region bounded by these surfaces, i.e., the causal region includes all points (x₁, x₂, x₃), for which the conditions

{(x₁)² + (x₂)² − (x₃)² = 0 and |x₃| ≤ cΔT} or{(x₁)² + (x₂)² = c²ΔT² and |x₃| ≤ cΔT} or{(x₁)² + (x₂)² − (x₃)² > 0 and (x₁)² + (x₂)² < c²ΔT²}

are fulfilled.

Theories with more than one dimension of time have sometimes been advanced in physics, whether as a serious description of reality or just as a curious possibility. Itzhak Bars's work on "two-time physics", inspired by the SO(10,2) symmetry of the extended supersymmetry structure of M-theory, is the most recent and systematic development of the concept (see also F-theory). Walter Craig and Steven Weinstein proved the existence of a well-posed initial value problem for the ultrahyperbolic equation (a wave equation in more than one time dimension). This showed that initial data on a mixed (spacelike and timelike) hypersurface obeying a particular nonlocal constraint evolves deterministically in the remaining time dimension.

Philosophy

An Experiment with Time by J. W. Dunne (1927) describes an ontology with an infinite hierarchy of time dimensions, inhabited by a similar hierarchy of selves. It was proposed as a solution to the problem of the passage of time. At the age of nine he had asked his nurse, was time the waypoints of yesterday, today and tomorrow, or was it the moment of "now" which travels from the one to the next? Dunne proposed that, in the context of a "block" spacetime as modelled by General Relativity, a second dimension of time was needed in order to measure the speed of one's progress along one's own timeline. This in turn required a level of the conscious self existing at the second level of time. But the same arguments then applied to this new level, requiring a third level, and so on in an infinite regress. At the end of the regress was a "superlative general observer" who existed in eternity. His infinite regress was criticised as logically flawed and unnecessary, although writers such as J. B. Priestley have acknowledged the possibility of his second time dimension.

Conceptual difficulties with multiple physical time dimensions have been raised in modern analytic philosophy.