 | ||
In music theory, a minor chord ( play D minor chord ) is a chord having a root, a minor third, and a perfect fifth. When a chord has these three notes alone, it is called a minor triad. Some minor triads with additional notes, such as the minor seventh chord, may also be called minor chords.
Contents
A minor triad can also be described as a minor third interval with a major third interval on top or as a root note, a note 3 semitones higher than the root, and a note 7 semitones higher than the root. Hence it can be represented by the integer notation {0, 3, 7}.
A major chord ( play ) differs from a minor chord in having a major third above the root instead of a minor third. It can also be described as a major third with a minor third on top, in contrast to a minor chord, which has a minor third with a major third on top. They both contain fifths, because a major third (4 semitones) plus a minor third (3 semitones) equals a fifth (7 semitones).
A diminished chord is a minor chord with a lowered fifth. play
An example of a minor chord is the C minor chord, which consists of the notes C (root), E♭ (minor third) and G (perfect fifth):
Play C minor chord in root position .The minor chord, along with the major chord, is one of the basic building blocks of tonal music and the common practice period. In Western music, a minor chord, in comparison, "sounds darker than a major chord" but is still considered highly consonant, stable, or as not requiring resolution.
Acoustic consonance of the minor chord
A unique particularity of the minor chord is that this is the only chord of three notes in which the three notes have one harmonic - hearable and with a not too high row - in common (more or less exactly, depending on the tuning system used) : This harmonic, common to the three notes, is situated 2 octaves above the high note of the chord : This is the harmonic of row 6 of the fundamental of the chord, the one of row 5 of middle note, the one of row 4 of the high note:
In the example do, mi♭, sol : a sol, 2 octaves above.Demonstration :
Just intonation
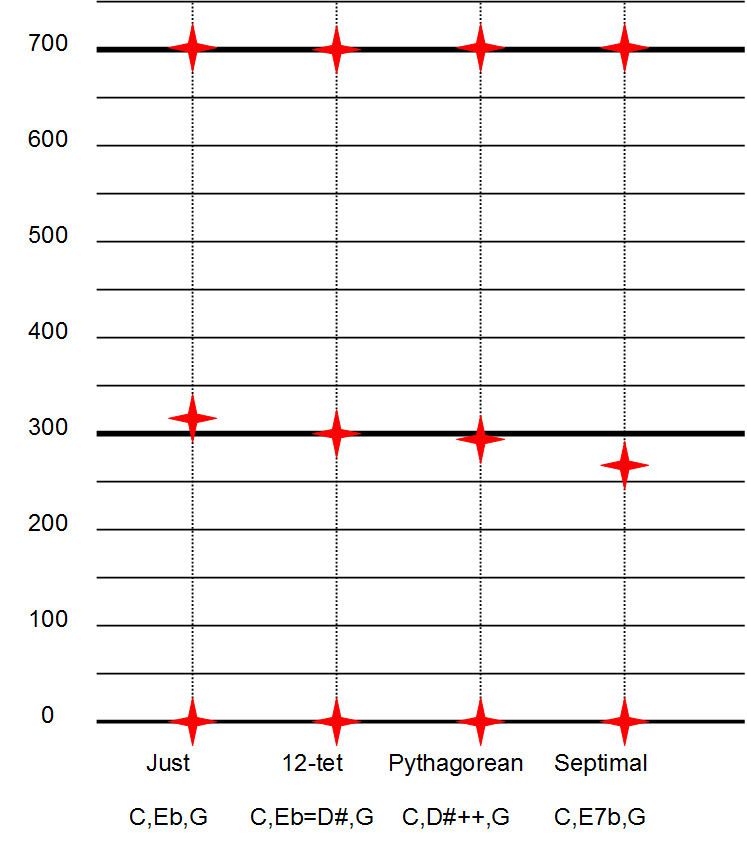
In just intonation, a minor chord is often (but not exclusively) tuned in the frequency ratio 10:12:15 ( play ). This is the first occurrence of a minor triad in the harmonic series (if on C: E-G-B). This may be found on iii, vi, ♭vi, ♭iii, and vii. In 12-TET, or twelve-tone equal temperament (now the most common tuning system in the West), a minor chord has 3 semitones between the root and third, 4 between the third and fifth, and 7 between the root and fifth. It is represented by the integer notation 0,3,7. The 12-TET fifth (700 cents) is only two cents narrower than the just perfect fifth (3:2, 701.9 cents), but the 12-TET minor third (300 cents) is noticeably (about 16 cents) narrower than the just minor third (6:5, 315.6 cents). The 12-TET minor third (300 cents) more closely approximates the 19-limit (Limit (music)) minor third 16:19 Play (297.5 cents, the nineteenth harmonic) with only 2 cents error. Ellis proposes that the conflict between mathematicians and physicists on one hand and practicing musicians on the other regarding the supposed inferiority of the minor chord and scale to the major may be explained due to physicists' comparison of just minor and major triads, in which case minor comes out the loser, versus the musicians' comparison of the equal tempered triads, in which case minor comes out the winner since the ET major third is 14 cents sharp from the just major third while the ET minor third closely approximates the consonant 19:16 minor third, which many find pleasing. Other just minor chord tunings include the supertonic triad in just intonation (27:32:40) the false minor triad, Play , 16:19:24 Play , 12:14:18 (6:7:9) Play (Septimal minor third), and the Pythagorean minor triad (54:64:81) Play . More tunings of the minor chord are also available in various equal temperaments other than 12-TET.
Rather than directly from the harmonic series, Sorge derived the minor chord from joining two major triads; for example the A minor triad being the confluence of the F and C major triads. A-C-E= f-A-C-E-g. Given justly tuned major triads this produces a justly tuned minor triad: 10:12:15 on 8/5.
