In mathematics, the McKay graph of a finite-dimensional representation V of a finite group G is a weighted quiver encoding the structure of the representation theory of G. Each node represents an irreducible representation of G. If χ i , χ j are irreducible representations of G then there is an arrow from χ i to χ j if and only if χ j is a constituent of the tensor product V ⊗ χ i . Then the weight nij of the arrow is the number of times this constituent appears in V ⊗ χ i . For finite subgroups H of GL(2, C), the McKay graph of H is the McKay graph of the canonical representation of H.
If G has n irreducible characters, then the Cartan matrix cV of the representation V of dimension d is defined by c V = ( d δ i j − n i j ) i j , where δ is the Kronecker delta. A result by Steinberg states that if g is a representative of a conjugacy class of G, then the vectors ( ( χ i ( g ) ) i are the eigenvectors of cV to the eigenvalues d − χ V ( g ) , where χ V is the character of the representation V.
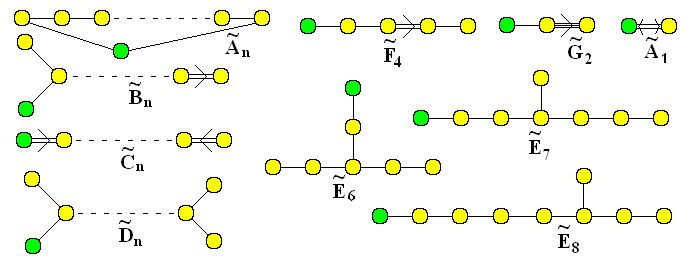
The McKay correspondence, named after John McKay, states that there is a one-to-one correspondence between the McKay graphs of the finite subgroups of SL(2, C) and the extended Dynkin diagrams, which appear in the ADE classification of the simple Lie Algebras.
Let G be a finite group, V be a representation of G and χ be its character. Let { χ 1 , … , χ d } be the irreducible representations of G. If
V ⊗ χ i = ∑ j n i j χ j , then define the McKay graph Γ G of G as follow:
To each irreducible representation of G corresponds a node in Γ G .There is an arrow from χ i to χ j if and only if nij > 0 and nij is the weight of the arrow: χ i → n i j χ j .If nij = nji, then we put an edge between χ i and χ j instead of a double arrow. Moreover, if nij = 1, then we do not write the weight of the corresponding arrow.We can calculate the value of nij by considering the inner product. We have the following formula:
n i j = ⟨ V ⊗ χ i , χ j ⟩ = 1 | G | ∑ g ∈ G V ( g ) χ i ( g ) χ j ( g ) ¯ , where ⟨ ⋅ , ⋅ ⟩ denotes the inner product of the characters.
The McKay graph of a finite subgroup of GL(2, C) is defined to be the McKay graph of its canonical representation.
For finite subgroups of SL(2, C), the canonical representation is self-dual, so nij = nji for all i, j. Thus, the McKay graph of finite subgroups of SL(2, C) is undirected.
In fact, by the McKay correspondence, there is a one-to-one correspondence between the finite subgroups of SL(2, C) and the extended Coxeter-Dynkin diagrams of type A-D-E.
We define the Cartan matrix cV of V as follow:
c V = ( d δ i j − n i j ) i j , where δ i j is the Kronecker delta.
If the representation V of a finite group G is faithful, then the McKay graph of V is connected.The McKay graph of a finite subgroup of SL(2, C) has no self-loops, that is, nii = 0 for all i.The weights of the arrows of the McKay graph of a finite subgroup of SL(2, C) are always less or equal than one.Suppose G = A × B, and there are canonical irreducible representations cA and cB of A and B respectively. If χ i , i = 1, ..., k, are the irreducible representations of A and ψ j , j = 1, ..., l, are the irreducible representations of B, then χ i × ψ j 1 ≤ i ≤ k , 1 ≤ j ≤ l are the irreducible representations of A × B , where χ i × ψ j ( a , b ) = χ i ( a ) ψ j ( b ) , ( a , b ) ∈ A × B . In this case, we have
⟨ ( c A × c B ) ⊗ ( χ i × ψ l ) , χ n × ψ p ⟩ = ⟨ c A ⊗ χ k , χ n ⟩ ⋅ ⟨ c B ⊗ ψ l , ψ p ⟩ . Therefore, there is an arrow in the McKay graph of G between χ i × ψ j and χ k × ψ l if and only if there is an arrow in the McKay graph of A between χ i and χ k and there is an arrow in the McKay graph of B between ψ j and ψ l . In this case, the weight on the arrow in the McKay graph of G is the product of the weights of the two corresponding arrows in the McKay graphs of A and B.
Felix Klein proved that the finite subgroups of SL(2, C) are the binary polyhedral groups. The McKay correspondence states that there is a one-to-one correspondence between the McKay graphs of these binary polyhedral groups and the extended Dynkin diagrams. For example, let T ¯ be the binary tetrahedral group. Every finite subgroup of SL(2, C) is conjugate to a finite subgroup of SU(2, C). Consider the matrices in SU(2, C): S = ( i 0 0 − i ) , V = ( 0 i i 0 ) , U = 1 2 ( ϵ ϵ 3 ϵ ϵ 7 ) , where ε is a primitive eighth root of unity. Then, T ¯ is generated by S, U, V. In fact, we have
T ¯ = { U k , S U k , V U k , S V U k | k = 0 , … , 5 } . The conjugacy classes of T ¯ are the following:
C 1 = { U 0 = I } , C 2 = { U 3 = − I } , C 3 = { ± S , ± V , ± S V } , C 4 = { U 2 , S U 2 , V U 2 , S V U 2 } , C 5 = { − U , S U , V U , S V U } , C 6 = { − U 2 , − S U 2 , − V U 2 , − S V U 2 } , C 7 = { U , − S U , − V U , − S V U } . The character table of T ¯ is
Here ω = e 2 π i / 3 . The canonical representation is represented by c. By using the inner product, we have that the McKay graph of T ¯ is the extended Coxeter-Dynkin diagram of type E ~ 6 .