In mathematical analysis, Lorentz spaces, introduced by George Lorentz in the 1950s, are generalisations of the more familiar L p spaces.
The Lorentz spaces are denoted by L p , q . Like the L p spaces, they are characterized by a norm (technically a quasinorm) that encodes information about the "size" of a function, just as the L p norm does. The two basic qualitative notions of "size" of a function are: how tall is graph of the function, and how spread out is it. The Lorentz norms provide tighter control over both qualities than the L p norms, by exponentially rescaling the measure in both the range ( p ) and the domain ( q ). The Lorentz norms, like the L p norms, are invariant under arbitrary rearrangements of the values of a function.
The Lorentz space on a measure space ( X , μ ) is the space of complex-valued measurable functions f on X such that the following quasinorm is finite
∥ f ∥ L p , q ( X , μ ) = p 1 q ∥ t μ { | f | ≥ t } 1 p ∥ L q ( R + , d t t ) where 0 < p < ∞ and 0 < q ≤ ∞ . Thus, when q < ∞ ,
∥ f ∥ L p , q ( X , μ ) = p 1 q ( ∫ 0 ∞ t q μ { x : | f ( x ) | ≥ t } q p d t t ) 1 q . and, when q = ∞ ,
∥ f ∥ L p , ∞ ( X , μ ) p = sup t > 0 ( t p μ { x : | f ( x ) | > t } ) . It is also conventional to set L ∞ , ∞ ( X , μ ) = L ∞ ( X , μ ) .
The quasinorm is invariant under rearranging the values of the function f , essentially by definition. In particular, given a complex-valued measurable function f defined on a measure space, ( X , μ ) , its decreasing rearrangement function, f ∗ : [ 0 , ∞ ) → [ 0 , ∞ ] can be defined as
f ∗ ( t ) = inf { α ∈ R + : d f ( α ) ≤ t } where d f is the so-called distribution function of f , given by
d f ( α ) = μ ( { x ∈ X : | f ( x ) | > α } ) . Here, for notational convenience, inf ∅ is defined to be ∞ .
The two functions | f | and f ∗ are equimeasurable, meaning that
μ ( { x ∈ X : | f ( x ) | > α } ) = λ ( { t > 0 : f ∗ ( t ) > α } ) , α > 0 , where λ is the Lebesgue measure on the real line. The related symmetric decreasing rearrangement function, which is also equimeasurable with f , would be defined on the real line by
R ∋ t ↦ 1 2 f ∗ ( | t | ) . Given these definitions, for 0 < p < ∞ and 0 < q ≤ ∞ , the Lorentz quasinorms are given by
∥ f ∥ L p , q = { ( ∫ 0 ∞ ( t 1 p f ∗ ( t ) ) q d t t ) 1 q q ∈ ( 0 , ∞ ) , sup t > 0 t 1 p f ∗ ( t ) q = ∞ . When ( X , μ ) = ( N , # ) (the counting measure on N ), the resulting Lorentz space is a sequence space. However, in this case it is convenient to use different notation.
for ( a n ) n = 1 ∞ ∈ R N (or C N in the complex case), let ∥ ( a n ) n = 1 ∞ ∥ p = ( ∑ n = 1 ∞ | a n | p ) 1 / p denote the p-norm for 1 ≤ p < ∞ and ∥ ( a n ) n = 1 ∞ ∥ ∞ = sup | a n | the ∞-norm. Denote by ℓ p the Banach space of all sequences with finite p-norm. Let c 0 the Banach space of all sequences satisfying lim | a n | = 0 , endowed with the ∞-norm. Denote by c 00 the normed space of all sequences with only finitely many nonzero entries. These spaces all play a role in the definition of the Lorentz sequence spaces d ( w , p ) below.
Let w = ( w n ) n = 1 ∞ ∈ c 0 ∖ ℓ 1 be a sequence of positive real numbers satisfying 1 = w 1 ≥ w 2 ≥ w 3 ⋯ , and define the norm ∥ ( a n ) ∥ d ( w , p ) = sup σ ∈ Π ∥ ( a σ ( n ) w n 1 / p ) n = 1 ∞ ∥ p . The Lorentz sequence space d ( w , p ) is defined as the Banach space of all sequences where this norm is finite. Equivalently, we can define d ( w , p ) as the completion of c 00 under ∥ ⋅ ∥ d ( w , p ) .
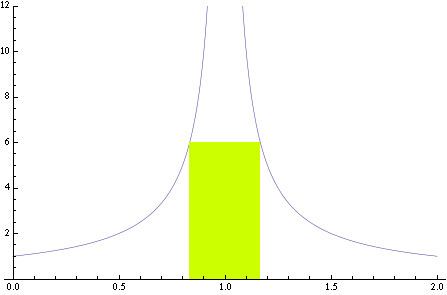
The Lorentz spaces are genuinely generalisations of the L p spaces in the sense that, for any p , L p , p = L p , which follows from Cavalieri's principle. Further, L p , ∞ coincides with weak L p . They are quasi-Banach spaces (that is, quasi-normed spaces which are also complete) and are normable for 1 < p < ∞ and 1 ≤ q ≤ ∞ . When p = 1 , L 1 , 1 = L 1 is equipped with a norm, but it is not possible to define a norm equivalent to the quasinorm of L 1 , ∞ , the weak L 1 space. As a concrete example that the triangle inequality fails in L 1 , ∞ , consider
f ( x ) = 1 x χ ( 0 , 1 ) ( x ) and g ( x ) = 1 1 − x χ ( 0 , 1 ) ( x ) , whose L 1 , ∞ quasi-norm equals one, whereas the quasi-norm of their sum f + g equals four.
The space L p , q is contained in L p , r whenever q < r . The Lorentz spaces are real interpolation spaces between L 1 and L ∞ .