Knaster–Kuratowski–Mazurkiewicz lemma

Statement

Let Δ n − 1 be a n − 1 -dimensional simplex with n vertices labeled as 1 , … , n .

A KKM covering is defined as a set C 1 , … , C n of closed sets such that for any I ⊆ { 1 , … , n } , the convex hull of the vertices corresponding to I is covered by ⋃ i ∈ I C i .

The KKM lemma says that a KKM covering has a non-empty intersection, i.e:

Example

The case n = 3 may serve as an illustration. In this case the simplex Δ 2 is a triangle, whose vertices we can label 1, 2 and 3. We are given three closed sets C 1 , C 2 , C 3 such that:

The KKM lemma states that the sets C 1 , C 2 , C 3 have at least one point in common.

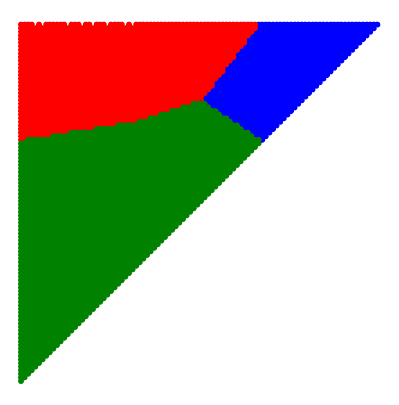
The lemma is illustrated by the picture on the right, in which set #1 is blue, set #2 is red and set #3 is green. The KKM requirements are satisfied, since:

The KKM lemma states that there is a point covered by all three colors simultaneously; such a point is clearly visible in the picture.

Equivalent results

There are several fixed-point theorems which come in three equivalent variants: an algebraic topology variant, a combinatorial variant and a set-covering variant. Each variant can be proved separately using totally different arguments, but each variant can also be reduced to the other variants in its row. Additionally, each result in the top row can be deduced from the one below it in the same column.

Permutations

David Gale proved the following generalization of the KKM lemma. Suppose that, instead of one KKM covering, we have n different KKM coverings: C 1 1 , … , C n 1 , … , C 1 n , … , C n n . Then, there exists a permutation π of the coverings with a non-empty intersection, i.e:

Gale wrote about his lemma: "A colloquial statement of this result is the red, white and blue lemma which asserts that if each of three people paint a triangle red, white and blue according to the KKM rules, then there will be a point which is in the red set of one person, the white set of another, the blue of the third".

Ravindra Bapat provided an alternative proof of this generalization, based on the permutation variant of Sperner's lemma.

Note: the original KKM lemma follows from the permutation lemma by simply picking n identical coverings.

Connecting-sets

A connecting set of a simplex is a connected set that touches all n faces of the simplex.

A generalized KKM covering is a covering C 1 , … , C n in which no C i contains a connecting set.

Any KKM-covering is a generalized-KKM-covering, since in a KKM covering, no C i even touches all n faces. However, there are generalized-KKM-coverings that are not KKM-coverings. An example is illustrated at the right. There, the red set touches all three faces, but it does not contain any connecting-set, since no connected component of it touches all three faces.

A theorem of Ravindra Bapat, generalizing Sperner's lemma, implies that the KKM lemma is true for generalized KKM coverings (he proved his theorem for n = 3 ).

The connecting-set variant also has a permutation variant, so that both these generalizations can be used simultaneously.

The KKMS theorem

The KKMS theorem is a generalization of the KKM lemma by Lloyd Shapley. It is useful in economics, especially in cooperative game theory.

While a KKM covering contains n sets, a KKMS covering contains 2 n − 1 sets - indexed by the nonempty subsets of { 1 , … , n } . For any I ⊆ { 1 , … , n } , the convex hull of the vertices corresponding to I should be covered by ⋃ J ⊆ I C J .

The KKMS theorem says that for any KKMS covering, there is a balanced collection B , such that the intersection of sets indexed by B is nonempty:

It remains to explain what a "balanced collection" is. A collection B of subsets of { 1 , … , n } is called balanced if, for each subset J ∈ B , we can select a weight w J ≥ 0 , such that, for each element x ∈ { 1 , … , n } , the sum of weights of all subsets that contain x is exactly 1. For example, suppose n = 3 . Then:

The KKMS theorem implies the KKM lemma. Suppose we have a KKM covering C i , for i = 1 , … , n . Construct a KKMS covering C J ′ as follows:

The KKM condition on the original covering C i implies the KKMS condition on the new covering C J ′ . Therefore, there exists a balanced collection such that the corresponding sets in the new covering have nonempty intersection. But the only possible balanced collection is the collection of all singletons; hence, the original covering has nonempty intersection.

The KKMS theorem has various proofs.

Reny and Holtz proved that the balanced set can also be chosen to be partnered.

Boundary conditions

Oleg R. Musin proved several generalizations of the KKM lemma and KKMS theorem, with boundary conditions on the coverings. The boundary conditions are related to homotopy.