Impulse excitation technique

Elastic properties

Different resonant frequencies can be excited dependent on the position of the support wires, the mechanical impulse and the microphone. The two most important resonant frequencies are the flexural which is controlled by the Young’s modulus of the sample and the torsional which is controlled by the shear modulus for isotropic materials.

For predefined shapes like rectangular bars, discs, rods and grinding wheels, dedicated software calculates the sample's elastic properties using the sample dimensions, weight and resonant frequency (ASTM E1876-15).

Flexure mode

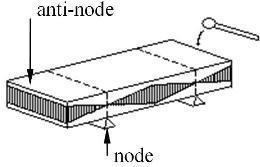
The first figure gives an example of a test-piece vibrating in the flexure mode. This induced vibration is also referred as the out-of-plane vibration mode. The in-plane vibration will be excited by turning the sample 90° on the axis parallel to its length. The natural frequency of this flexural vibration mode is characteristic for the dynamic Young's modulus. To minimize the damping of the test-piece, it has to be supported at the nodes where the vibration amplitude is zero. The test-piece is mechanically excited at one of the anti-nodes to cause maximum vibration.

Torsion mode

The second figure gives an example of a test-piece vibrating in the torsion mode. The natural frequency of this vibration is characteristic for the shear modulus. To minimize the damping of the test-piece, it has to be supported at the center of both axis. The mechanical excitation has to be performed in one corner in order to twist the beam rather than flexing it.

Poisson's ratio

The Poisson’s ratio is a measure in which a material tends to expand in directions perpendicular to the direction of compression. After measuring the Young's modulus and the shear modulus, dedicated software determines the Poisson’s ratio using Hooke’s law which can only be applied to isotropic materials according to the different standards.

Internal friction / Damping

Material damping or internal friction is characterized by the decay of the vibration amplitude of the sample in free vibration as the logarithmic decrement. The damping behaviour originates from anelastical processes occurring in a strained solid i.e. thermoelastic damping, magnetic damping, viscous damping, defect damping,… For example, different materials defects (dislocations, vacancies,..) can contribute to an increase in the internal friction between the vibrating defects and the neighboring regions.

Dynamic vs. static methods

Considering the importance of elastic properties for design and engineering applications, a number of experimental techniques are developed and these can be classified into 2 groups; static and dynamic methods. Statics methods (like the four-point bending test and nanoindentation) are based on direct measurements of stresses and strains during mechanical tests. Dynamic methods (like ultrasound spectroscopy and impulse excitation technique) provide an advantage over static methods because the measurements are relatively quick and simple and involve small elastic strains. Therefore, IET is very suitable for porous and brittle materials like ceramics, refractories,… The technique can also be easily modified for high temperature experiments and only a small amount of material needs to be available.

Accuracy and uncertainty

The most important parameters to define the measurement uncertainty are the mass and dimensions of the sample. Therefore, each parameter has to be measured (and prepared) to a level of accuracy of 0.1%. Especially, the sample thickness is most critical (third power in the equation for Young’s modulus). In that case, an overall accuracy of 1% can be obtained practically in most applications.

Applications

The impulse excitation technique can be used in a wide range of applications. Nowadays, IET equipment can perform measurements between -50 °C and 1700 °C in different atmospheres (air, inert, vacuum). IET is mostly used in research and as quality control tool to study the transitions as function of time and temperature. A detailed insight into the material crystal structure can be obtained by studying the elastic and damping properties. For example, the interaction of dislocations and point defects in carbon steels are studied. Also the material damage accumulated during a thermal shock treatment can be determined for refractory materials. This can be an advantage in understanding the physical properties of certain materials. Finally, the technique can be used to check the quality of systems. In this case, a reference piece is required to obtain a reference frequency spectrum. Engine blocks for example can be tested by tapping them and comparing the recorded signal with a pre-recorded signal of a reference engine block.

Young’s modulus

E = 0.9465 ( m f f 2 b ) ( L 3 t 3 ) T

with

T = 1 + 6.585 ( t L ) 2 E the Young's modulus m the mass f_f the flexural frequency b the width L the length t the thickness T the correction factor The correction factor can only be used if L/t ≥ 20!

Shear modulus

G = 4 L m f t 2 b t R

with

R = [ 1 + ( b t ) 2 4 − 2.521 t b ( 1 − 1.991 e π b t + 1 ) ] [ 1 + 0.00851 b 2 L 2 ] − 0.060 ( b L ) 3 2 ( b t − 1 ) 2 Note that we assume that b≥t

G the shear modulus

f_t the torsional frequency m the mass b the width L the length t the thickness R the correction factor

Young’s modulus

E = 1.6067 ( L 3 d 4 ) m f f 2 T ′

with

T ′ = 1 + 4.939 ( d L ) 2 E the Young's modulus m the mass f_f the flexural frequency d the diameter L the length T' the correction factor The correction factor can only be used if L/d ≥ 20!

Shear modulus

G = 16 ( L π d 2 ) m f t 2

with

f_t the torsional frequency m the mass d the diameter L the length

Poisson ratio

If the Young's modulus and shear modulus are known, the Poisson's ratio can be calculated according to:

ν = ( E 2 G ) − 1

Damping coefficient

The induced vibration signal (in the time domain) is fitted as a sum of exponentially damped sinusoidal functions according to:

x ( t ) = ∑ A e − k t sin ⁡ ( 2 π f t + ϕ )

with

f the natural frequency δ = kt the logarithmic decrement In this case, the damping parameter Q⁻¹ can be defined as: Q − 1 = Δ W 2 π W = k π f with W the energy of the system

Standards

ASTM E1876 - 15 Standard Test Method for Dynamic Youngs Modulus, Shear Modulus, and Poissons Ratio by Impulse Excitation of Vibration. www.astm.org.

ISO 12680-1:2005 - Methods of test for refractory products -- Part 1: Determination of dynamic Young's modulus (MOE) by impulse excitation of vibration. ISO.

DIN EN 843-2:2007 Advanced technical ceramics - Mechanical properties of monolithic ceramics at room temperature". webstore.ansi.org.