 | ||
In geometry, a flexible polyhedron is a polyhedral surface that allows continuous non-rigid deformations such that all faces remain rigid. The Cauchy rigidity theorem shows that in dimension 3 such a polyhedron cannot be convex (this is also true in higher dimensions).
Contents
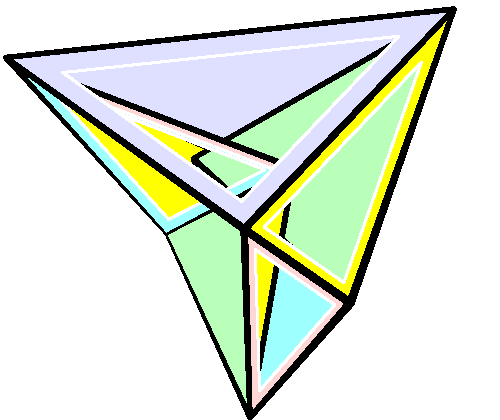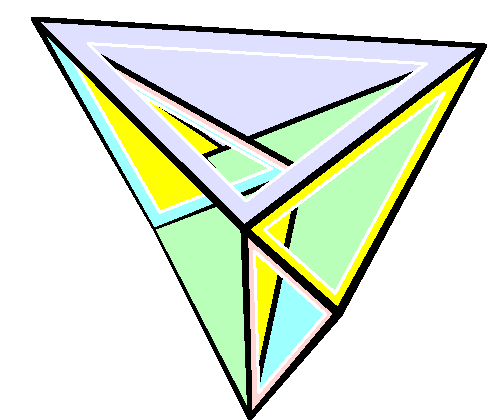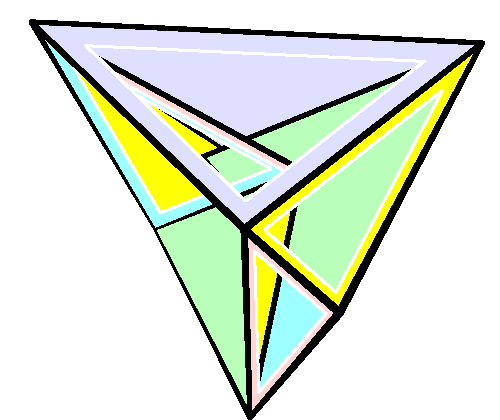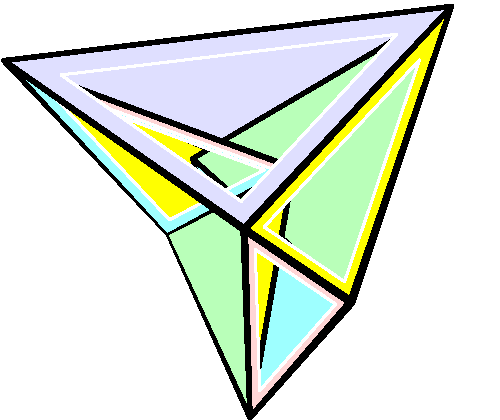
The first examples of flexible polyhedra, now called Bricard's octahedra, were discovered by Raoul Bricard (1897). They are self-intersecting surfaces isometric to an octahedron. The first example of a flexible non-self-intersecting surface in R3, the Connelly sphere, was discovered by Robert Connelly (1977).
Bellows conjecture
In the late 1970s Connelly and D. Sullivan formulated the bellows conjecture stating that the volume of a flexible polyhedron is invariant under flexing. This conjecture was proved for polyhedra homeomorphic to a sphere by I. Kh. Sabitov (1995) using elimination theory, and then proved for general orientable 2-dimensional polyhedral surfaces by Robert Connelly, I. Sabitov, and Anke Walz (1997). The proof extends Piero della Francesca's formula for the volume of a tetrahedron to a formula for the volume of any polyhedron. The extended formula shows that the volume must be a root of a polynomial whose coefficients depend only on the lengths of the polyhedron's edges. Since the edge lengths cannot change as the polyhedron flexes, the volume must remain at one of the finitely many roots of the polynomial, rather than changing continuously (Demaine & O'Rourke 2007).
Scissor congruence
Connelly conjectured that the Dehn invariant of a flexible polyhedron is invariant under flexing. This is known as the strong bellows conjecture. Preservation of the Dehn invariant is known to be equivalent to scissors congruence of the enclosed region under flexing. It is known that the Bricard octahedra obey the strong bellows conjecture, as does Steffen's polyhedron, a non-self-intersecting flexible polyhedron derived from them (Alexandrov 2010). The total mean curvature of a flexible polyhedron, defined as the sum of the products of edge lengths with exterior dihedral angles, is a function of the Dehn invariant that is also known to stay constant while a polyhedron flexes (Alexander 1985). However, the full strong bellows conjecture remains unproven.
Generalizations
Flexible 4-polytopes in 4-dimensional Euclidean space and 3-dimensional hyperbolic space were studied by Hellmuth Stachel (2000). In dimensions
