Samiksha Jaiswal (Editor)

Champernowne constant

Updated on
Edit
Like
Comment
Share on FacebookTweet on TwitterShare on LinkedInShare on Reddit
Champernowne constant

In mathematics, the Champernowne constant C10 is a transcendental real constant whose decimal expansion has important properties. It is named after economist and mathematician D. G. Champernowne, who published it as an undergraduate in 1933.

Contents

For base 10, the number is defined by concatenating representations of successive integers:

C10 = 0.12345678910111213141516…  (sequence A033307 in the OEIS).

Champernowne constants can also be constructed in other bases, similarly, for example:

C2 = 0.11011100101110111… 2C3 = 0.12101112202122… 3.

The Champernowne constant can be expressed exactly as an infinite series:

C 10 = ∑ n = 1 ∞ ∑ k = 10 n − 1 10 n − 1 k 10 n ( k − 10 n − 1 + 1 ) + 9 ∑ ℓ = 1 n − 1 10 ℓ − 1 ℓ

and this series generalizes to arbitrary bases b by replacing 10 and 9 with b and b − 1 respectively.

The Champernowne word or Barbier word is the sequence of digits of Ck.

Normality

A real number x is said to be normal if its digits in every base follow a uniform distribution: all digits being equally likely, all pairs of digits equally likely, all triplets of digits equally likely, etc. x is said to be normal in base b if its digits in base b follow a uniform distribution.

If we denote a digit string as [a0,a1,...], then, in base ten, we would expect strings [0],[1],[2],...,[9] to occur 1/10 of the time, strings [0,0],[0,1],...,[9,8],[9,9] to occur 1/100 of the time, and so on, in a normal number.

Champernowne proved that C 10 is normal in base ten, although it is possible that it is not normal in other bases.

Continued fraction expansion

The simple continued fraction expansion of Champernowne's constant has been studied as well. Kurt Mahler showed that the constant is transcendental; therefore its continued fraction does not terminate (because it is not rational) and is aperiodic (because it is not an irreducible quadratic).

The terms in the continued fraction expansion exhibit very erratic behaviour, with huge terms appearing between many small ones. For example, in base 10,

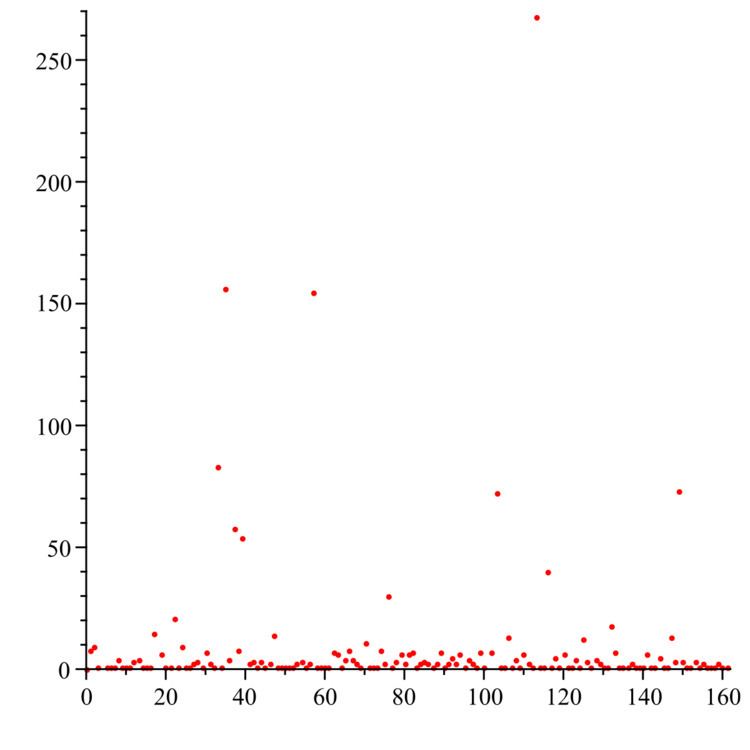
C10 = [0; 8, 9, 1, 149083, 1, 1, 1, 4, 1, 1, 1, 3, 4, 1, 1, 1, 15,

The large number at position 19 has 166 digits, and the next very large term at position 41 of the continued fraction has 2504 digits. The fact that there are such large numbers as terms of the continued fraction expansion is equivalent to saying that the convergents obtained by stopping before these large numbers provide an exceptionally good approximation of the Champernowne constant.

It can be understood from infinite series expression of C 10 : for a specified n we can always approximate the sum over k by setting the upper limit to ∞ instead of 10 n − 1 . Then we ignore the terms for higher n .

For example, if we keep lowest order of n, it is equivalent to truncating before the 4th partial quotient, we obtain the partial sum

10 / 81 = ∑ n = 1 ∞ n / 10 n = 0. 123456790 ¯

which approximates Champernowne's constant with an error of about 1 × 10−9. While truncating just before the 18th partial quotient, we get the approximation to second order:

60499999499 490050000000 = 0.123456789 + 10 − 9 ∑ k = 10 ∞ k / 10 2 ( k − 9 ) = 0.123456789 + 10 − 9 991 9801 = 0.123456789 101112 … 96979900010203040506070809 ¯ ,

which approximates Champernowne's constant with error approximately 9 × 10−190.

Irrationality measure

The irrationality measure of C 10 is μ ( C 10 ) = 10 , and more generally μ ( C b ) = b for any base b ≥ 2 .

References

Champernowne constant Wikipedia
(Text) CC BY-SA


Similar Topics