 | ||
In 8-dimensional geometry, the 142 is a uniform 8-polytope, constructed within the symmetry of the E8 group.
Contents
Its Coxeter symbol is 142, describing its bifurcating Coxeter-Dynkin diagram, with a single ring on the end of the 1-node sequences.
The rectified 142 is constructed by points at the mid-edges of the 142 and is the same as the birectified 241, and the quadrirectified 421.
These polytopes are part of a family of 255 (28 − 1) convex uniform polytopes in 8-dimensions, made of uniform polytope facets and vertex figures, defined by all permutations of rings in this Coxeter-Dynkin diagram: .
142 polytope
The 142 is composed of 2400 facets: 240 132 polytopes, and 2160 7-demicubes (141). Its vertex figure is a birectified 7-simplex.
This polytope, along with the demiocteract, can tessellate 8-dimensional space, represented by the symbol 152, and Coxeter-Dynkin diagram: .
Alternate names
Coordinates
The 17280 vertices can be defined as sign and location permutations of:
All sign combinations (32): (280×32=8960 vertices)
(4, 2, 2, 2, 2, 0, 0, 0)Half of the sign combinations (128): ((1+8+56)×128=8320 vertices)
(2, 2, 2, 2, 2, 2, 2, 2)(5, 1, 1, 1, 1, 1, 1, 1)(3, 3, 3, 1, 1, 1, 1, 1)The edge length is 2√2 in this coordinate set, and the polytope radius is 4√2.
Construction
It is created by a Wythoff construction upon a set of 8 hyperplane mirrors in 8-dimensional space.
The facet information can be extracted from its Coxeter-Dynkin diagram: .
Removing the node on the end of the 2-length branch leaves the 7-demicube, 141, .
Removing the node on the end of the 4-length branch leaves the 132, .
The vertex figure is determined by removing the ringed node and ringing the neighboring node. This makes the birectified 7-simplex, 042, .
Projections
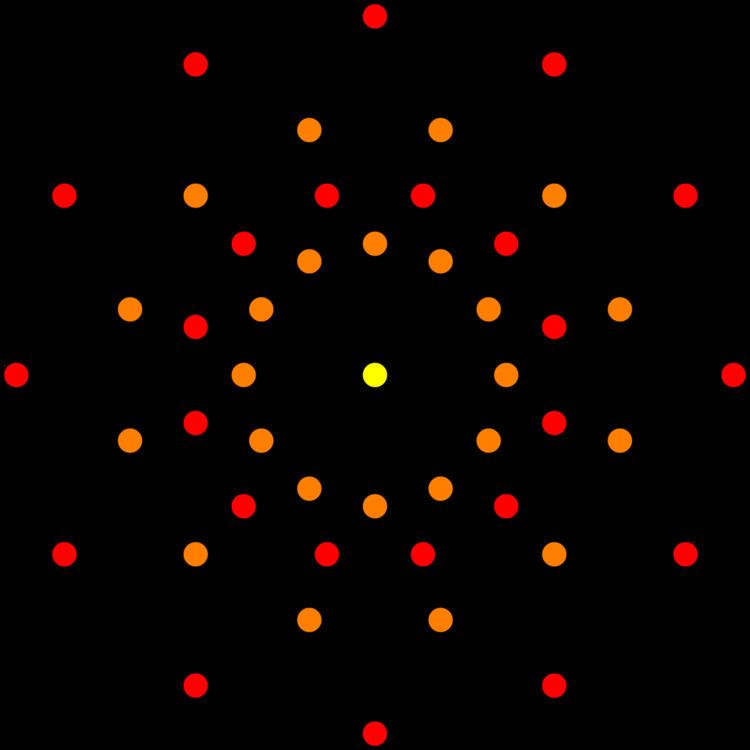
Orthographic projections are shown for the sub-symmetries of E8: E7, E6, B8, B7, B6, B5, B4, B3, B2, A7, and A5 Coxeter planes, as well as two more symmetry planes of order 20 and 24. Vertices are shown as circles, colored by their order of overlap in each projective plane.
Rectified 142 polytope
The rectified 142 is named from being a rectification of the 142 polytope, with vertices positioned at the mid-edges of the 142.
Alternate names
Construction
It is created by a Wythoff construction upon a set of 8 hyperplane mirrors in 8-dimensional space.
The facet information can be extracted from its Coxeter-Dynkin diagram: .
Removing the node on the end of the 1-length branch leaves the birectified 7-simplex,
Removing the node on the end of the 2-length branch leaves the 7-demicube, 141, .
Removing the node on the end of the 3-length branch leaves the 132, .
The vertex figure is determined by removing the ringed node and ringing the neighboring node. This makes the 5-cell-triangle duoprism prism, .
Projections
Orthographic projections are shown for the sub-symmetries of B6, B5, B4, B3, B2, A7, and A5 Coxeter planes. Vertices are shown as circles, colored by their order of overlap in each projective plane.
(Planes for E8: E7, E6, B8, B7, [20], [24] are not shown for being too large to display.)
