 | ||
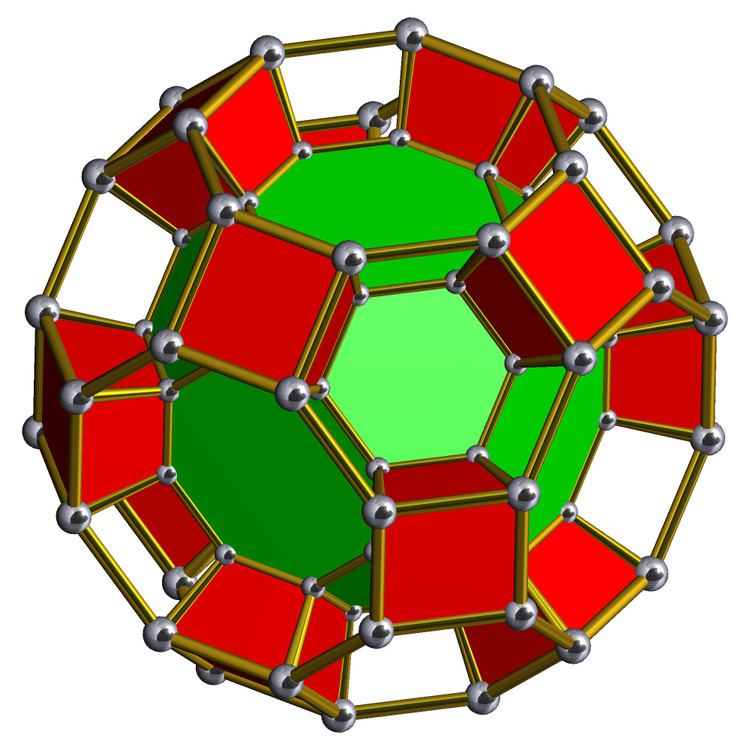
In geometry, a truncated cuboctahedral prism or great rhombicuboctahedral prism is a convex uniform polychoron (four-dimensional polytope).
Contents
It is one of 18 convex uniform polyhedral prisms created by using uniform prisms to connect pairs of Platonic solids or Archimedean solids in parallel hyperplanes.
Net
Alternative names
Related polytopes
A full snub cubic antiprism or omnisnub cubic antiprism can be defined as an alternation of an truncated cuboctahedral prism, represented by ht0,1,2,3{4,3,2}, or , although it cannot be constructed as a uniform polychoron. It has 76 cells: 2 snub cubes connected by 12 tetrahedrons, 6 square antiprisms, and 8 octahedrons, with 48 tetrahedrons in the alternated gaps. There are 48 vertices, 192 edges, and 220 faces (12 squares, and 16+192 triangles). It has [4,3,2]+ symmetry, order 48.
Vertex figure for full snub cuboctahedral antiprism
