Trisha Shetty (Editor)

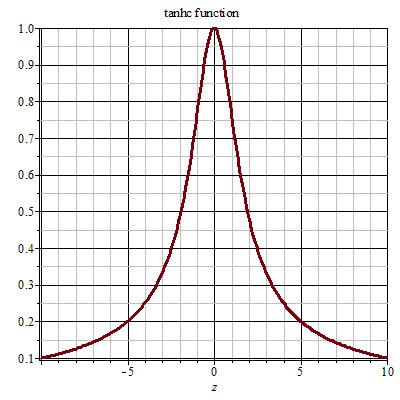
Tanhc function

Updated on
Edit
Like
Comment
Share on FacebookTweet on TwitterShare on LinkedInShare on Reddit
Tanhc function

In mathematics, the tanhc function is defined as

Contents

tanhc ⁡ ( z ) = tanh ⁡ ( z ) z
Imaginary part in complex plane
  • Im ⁡ ( tanh ⁡ ( x + i y ) x + i y )
    • Real part in complex plane
  • Re ⁡ ( tanh ⁡ ( x + i y ) x + i y )
    • absolute magnitude
  • | tanh ⁡ ( x + i y ) x + i y |
    • First-order derivative
  • 1 − tanh ⁡ ( z ) ) 2 z − tanh ⁡ ( z ) z 2
    • Real part of derivative
  • − Re ⁡ ( − 1 − ( tanh ⁡ ( x + i y ) ) 2 x + i y + tanh ⁡ ( x + i y ) ( x + i y ) 2 )
    • Imaginary part of derivative
  • − Im ⁡ ( − 1 − ( tanh ⁡ ( x + i y ) ) 2 x + i y + tanh ⁡ ( x + i y ) ( x + i y ) 2 )
    • absolute value of derivative
  • | − 1 − ( tanh ⁡ ( x + i y ) ) 2 x + i y + tanh ⁡ ( x + i y ) ( x + i y ) 2 |

    • In terms of other special functions

  • tanhc ⁡ ( z ) = 2 K u m m e r M ( 1 , 2 , 2 z ) ( 2 i z + π ) K u m m e r M ( 1 , 2 , i π − 2 z ) e 2 z − 1 / 2 i π
  • tanhc ⁡ ( z ) = 2 HeunB ⁡ ( 2 , 0 , 0 , 0 , 2 z ) ( 2 i z + π ) HeunB ⁡ ( 2 , 0 , 0 , 0 , 2 1 / 2 i π − z ) e 2 z − 1 / 2 i π
  • tanhc ⁡ ( z ) = i   W h i t t a k e r M ( 0 , 1 / 2 , 2 z ) W h i t t a k e r M ( 0 , 1 / 2 , i π − 2 z ) z

    • Series expansion

    tanhc ⁡ z ≈ ( 1 − 1 3 z 2 + 2 15 z 4 − 17 315 z 6 + 62 2835 z 8 − 1382 155925 z 10 + 21844 6081075 z 12 − 929569 638512875 z 14 + O ( z 16 ) ) ∫ 0 z tanh ⁡ ( x ) x d x = ( z − 1 9 z 3 + 2 75 z 5 − 17 2205 z 7 + 62 25515 z 9 − 1382 1715175 z 11 + O ( z 13 ) )

    Pade approximation

    T a n h c ( z ) = ( 1 + 7 51 z 2 + 1 255 z 4 + 2 69615 z 6 + 1 34459425 z 8 ) ( 1 + 8 17 z 2 + 7 255 z 4 + 4 9945 z 6 + 1 765765 z 8 ) − 1

    References

    Tanhc function Wikipedia
    (Text) CC BY-SA


    Similar Topics