 | ||
In projective geometry an ovoid is a sphere like pointset (surface) in a projective space of dimension d ≥ 3. Simple examples in a real projective space are hyperspheres (quadrics). The essential geometric properties of an ovoid
Contents
- Definition of an ovoid
- In real projective space inhomogeneous representation
- Finite examples
- Criteria for an ovoid to be a quadric
- Generalization semi ovoid
- References
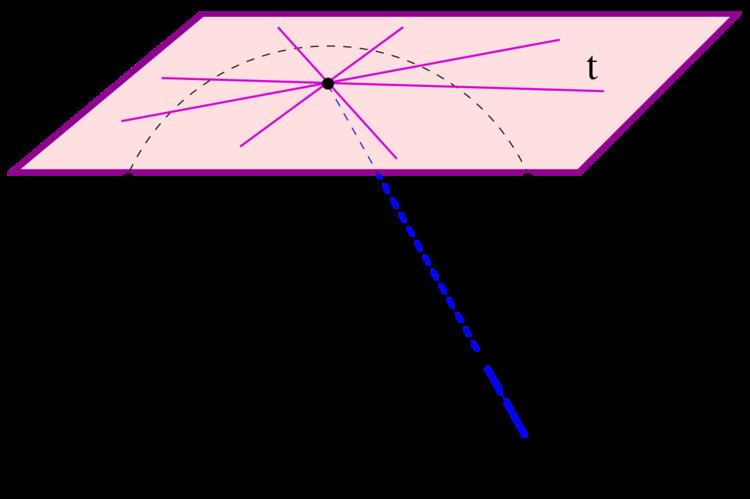
- Any line intersects
O in at most 2 points, - The tangents at a point cover a hyperplane (and nothing more), and
-
O contains no lines.
Property 2) excludes degenerated cases (cones,...). Property 3) excludes ruled surfaces (hyperboloids of one sheet, ...).
An ovoid is the spatial analog of an oval in a projective plane.
An ovoid is a special type of a quadratic set
Ovoids play an essential role in constructing examples of Möbius planes and higher dimensional Möbius geometries.
Definition of an ovoid
In the case of
From the viewpoint of the hyperplane sections, an ovoid is a rather homogeneous object, because
For finite projective spaces of dimension d ≥ 3 (i.e., the point set is finite, the space is pappian), the following result is true:
Replacing the word projective in the definition of an ovoid by affine, gives the definition of an affine ovoid.
If for an (projective) ovoid there is a suitable hyperplane
In real projective space (inhomogeneous representation)
-
O = { ( x 1 , . . . , x d ) ∈ R d | x 1 2 + ⋯ + x d 2 = 1 } , (hypersphere) -
O = { ( x 1 , . . . , x d ) ∈ R d | x d = x 1 2 + ⋯ + x d − 1 2 } ∪ { point at infinity of x d -axis }
These two examples are quadrics and are projectively equivalent.
Simple examples, which are not quadrics can be obtained by the following constructions:
(a) Glue one half of a hypersphere to a suitable hyperellipsoid in a smooth way.(b) In the first two examples replace the expression x12 by x14.Remark: The real examples can not be converted into the complex case (projective space over
But the following method guarantees many non quadric ovoids:
Finite examples
The last result can not be extended to even characteristic, because of the following non-quadric examples:
the pointset
Criteria for an ovoid to be a quadric
An ovoidal quadric has many symmetries. In particular:
In the finite case one gets from Segre's theorem:
Generalization: semi ovoid
Removing condition (1) from the definition of an ovoid results in the definition of a semi-ovoid:
A point setthe following conditions hold:
(SO1) For any pointA semi ovoid is a special semi-quadratic set which is a generalization of a quadratic set. The essential difference between a semi-quadratic set and a quadratic set is the fact, that there can be lines which have 3 points in common with the set and the lines are not contained in the set.
Examples of semi-ovoids are the sets of isotropic points of an hermitian form. They are called hermitian quadrics.
As for ovoids in literature there are criteria, which make a semi-ovoid to a hemitian quadric. (for example)
Semi-ovoids are used in the construction of examples of Möbius geometries.
