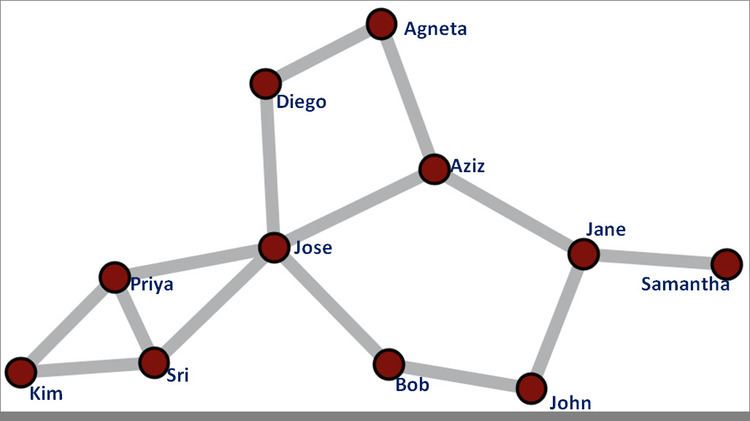
 | ||
In graph theory, the Katz centrality of a node is a measure of centrality in a network. It was introduced by Leo Katz in 1953 and is used to measure the relative degree of influence of an actor (or node) within a social network. Unlike typical centrality measures which consider only the shortest path (the geodesic) between a pair of actors, Katz centrality measures influence by taking into account the total number of walks between a pair of actors.
Contents
It is similar to Google's PageRank and to the eigenvector centrality.
Measuring Katz centrality
Katz centrality computes the relative influence of a node within a network by measuring the number of the immediate neighbors (first degree nodes) and also all other nodes in the network that connect to the node under consideration through these immediate neighbors. Connections made with distant neighbors are, however, penalized by an attenuation factor
For example, in the figure on the right, assume that John’s centrality is being measured and that
Mathematical formulation
Let A be the adjacency matrix of a network under consideration. Elements
Note that the above definition uses the fact that the element at location
Here
Applications
Katz centrality can be used to compute centrality in directed networks such as citation networks and the World Wide Web. Katz centrality is more suitable in the analysis of directed acyclic graphs where traditionally used measures like eigenvector centrality are rendered useless. Katz centrality can also be used in estimating the relative status or influence of actors in a social network. In neuroscience, it is found that Katz centrality correlates with the relative firing rate of neurons in a neural network.
