 | ||
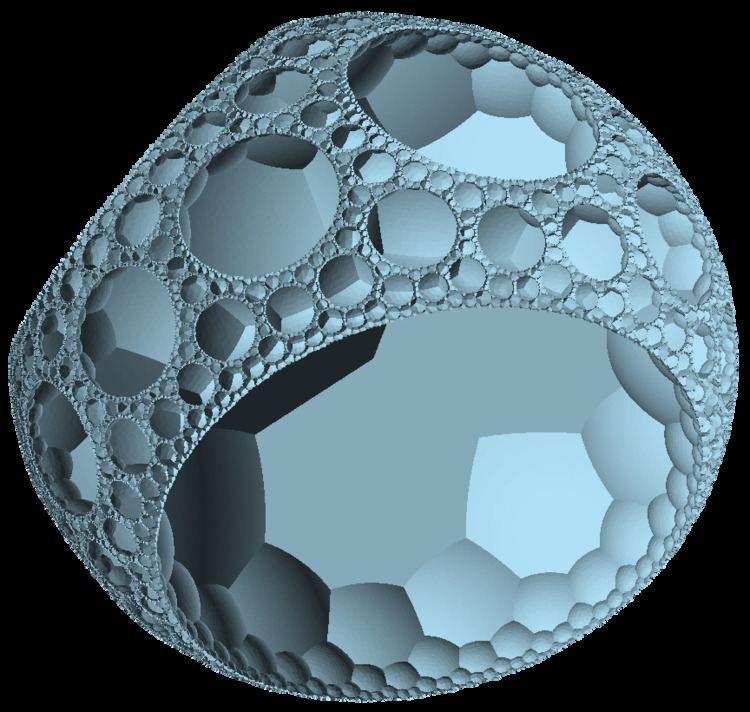
In the geometry of hyperbolic 3-space, the heptagonal tiling honeycomb a regular space-filling tessellation (or honeycomb). Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
The Schläfli symbol of the heptagonal tiling honeycomb is {7,3,3}, with three heptagonal tilings meeting at each edge. The vertex figure of this honeycomb is an tetrahedron, {3,3}.
Related polytopes and honeycombs
It is a part of a series of regular polytopes and honeycombs with {p,3,3} Schläfli symbol, and tetrahedral vertex figures:
References
Heptagonal tiling honeycomb Wikipedia(Text) CC BY-SA
