Edges 12 Vertex configuration 3.4.3.4 | Vertices 6 Schläfli symbol r{3,4}/2 or r{3,4}3 | |
 | ||
Type abstract polyhedron
globally projective polyhedron Faces 7:
4 triangles
3 squares | ||
A hemi-cuboctahedron is an abstract polyhedron, containing half the faces of a semiregular cuboctahedron.
Contents
It has 4 triangular faces and 3 square faces, 12 edges, and 6 vertices. It can be seen as a rectified hemi-octahedron or rectified hemi-cube.
It can be realized as a projective polyhedron (a tessellation of the real projective plane by 4 triangles and 3 square), which can be visualized by constructing the projective plane as a hemisphere where opposite points along the boundary are connected.
Dual
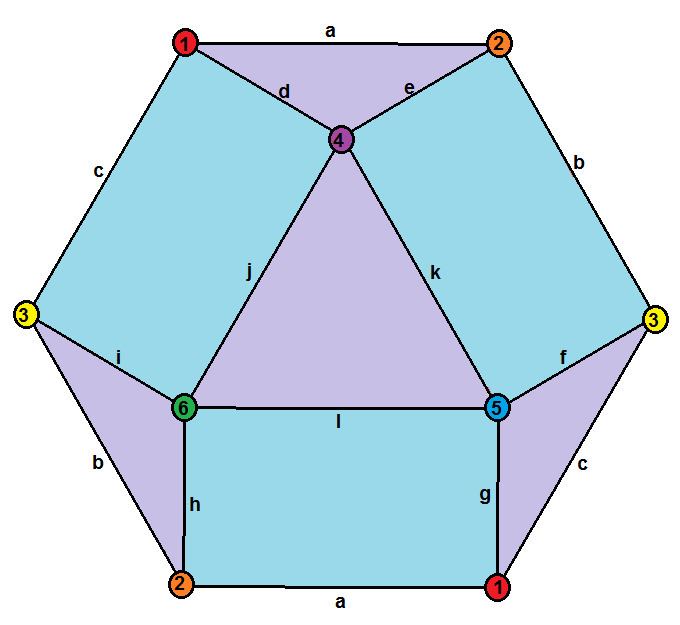
Its dual polyhedron is a rhombic hemi-dodecahedron which has 7 vertices (1-7), 12 edges (a-l), and 6 rhombic faces (A-F).
Related polyhedra
It has a real presentation as a uniform star polyhedron, the tetrahemihexahedron.
References
Hemi-cuboctahedron Wikipedia(Text) CC BY-SA