 | ||
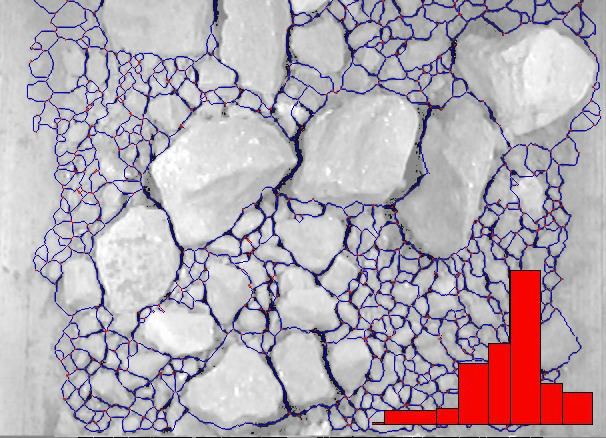
In mathematical morphology, granulometry is an approach to compute a size distribution of grains in binary images, using a series of morphological opening operations. It was introduced by Georges Matheron in the 1960s, and is the basis for the characterization of the concept of size in mathematical morphology.
Contents
Granulometry generated by a structuring element
Let B be a structuring element in an Euclidean space or grid E, and consider the family
where
Let X be a set (i.e., a binary image in mathematical morphology), and consider the series of sets
where
The granulometry function
The pattern spectrum or size distribution of X is the collection of sets
The parameter k is referred to as size, and the component k of the pattern spectrum
Sieving axioms
The above common method is a particular case of the more general approach derived by Matheron.
The French mathematician was inspired by sieving as a means of characterizing size. In sieving, a granular sample is worked through a series of sieves with decreasing hole sizes. As a consequence, the different grains in the sample are separated according to their sizes.
The operation of passing a sample through a sieve of certain hole size "k" can be mathematically described as an operator
- Anti-extensivity: Each sieve reduces the amount of grains, i.e.,
Ψ k ( X ) ⊆ X , - Increasingness: The result of sieving a subset of a sample is a subset of the sieving of that sample, i.e.,
X ⊆ Y ⇒ Ψ k ( X ) ⊆ Ψ k ( Y ) , - "Stability": The result of passing through two sieves is determined by the sieve with smallest hole size. I.e.,
Ψ k Ψ m ( X ) = Ψ m Ψ k ( X ) = Ψ max ( k , m ) ( X ) .
A granulometry-generating family of operators should satisfy the above three axioms.
In the above case (granulometry generated by a structuring element),
Another example of granulometry-generating family is when
