Feynman slash notation ~ Everything You Need to Know with Photos

QFT12.5 Feynman notation

In the study of Dirac fields in quantum field theory, Richard Feynman invented the convenient Feynman slash notation (less commonly known as the Dirac slash notation). If A is a covariant vector (i.e., a 1-form),

Identities

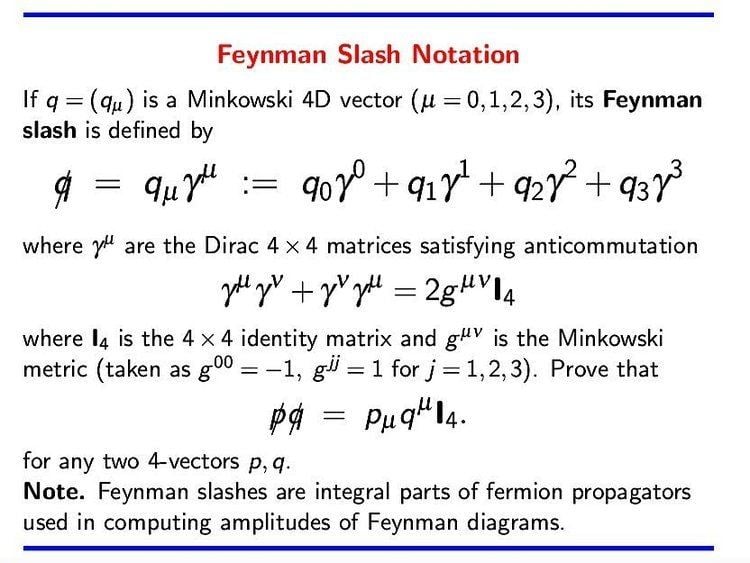
Sam Walters âï¸ on Twitter: "Richard #Feynman introduced his slash notation for vectors since they would often appear "dotted" together with #Dirac matrices in #quantum electrodynamics calculations. The slash of a vector

Using the anticommutators of the Gamma matrices, one can show that for any a μ and b μ ,

a / a / ≡ a μ a μ ⋅ I 4 = a 2 ⋅ I 4 a / b / + b / a / ≡ 2 a ⋅ b ⋅ I 4 .

where I 4 is the identity matrix in four dimensions.

In particular,

∂ / 2 ≡ ∂ 2 ⋅ I 4 .

Further identities can be read off directly from the gamma matrix identities by replacing the metric tensor with inner products. For example,

tr ⁡ ( a / b / ) ≡ 4 a ⋅ b tr ⁡ ( a / b / c / d / ) ≡ 4 [ ( a ⋅ b ) ( c ⋅ d ) − ( a ⋅ c ) ( b ⋅ d ) + ( a ⋅ d ) ( b ⋅ c ) ] tr ⁡ ( γ 5 a / b / c / d / ) ≡ 4 i ϵ μ ν λ σ a μ b ν c λ d σ γ μ a / γ μ ≡ − 2 a / γ μ a / b / γ μ ≡ 4 a ⋅ b ⋅ I 4 γ μ a / b / c / γ μ ≡ − 2 c / b / a /

where

ϵ μ ν λ σ is the Levi-Civita symbol.

With four-momentum

Often, when using the Dirac equation and solving for cross sections, one finds the slash notation used on four-momentum: using the Dirac basis for the gamma matrices,

γ 0 = ( I 0 0 − I ) , γ i = ( 0 σ i − σ i 0 )

as well as the definition of four momentum,

p μ = ( E , − p x , − p y , − p z )

we see explicitly that

p / = γ μ p μ = γ 0 p 0 + γ i p i = [ p 0 0 0 − p 0 ] + [ 0 σ i p i − σ i p i 0 ] = [ E − σ ⋅ p → σ ⋅ p → − E ] .

Similar results hold in other bases, such as the Weyl basis.

References

Feynman slash notation Wikipedia

(Text) CC BY-SA

QFT12.5 Feynman notation

Contents

Identities

With four-momentum

References