Edges 36 Vertex configuration 6(3.4.3.4)12(3.4) | Vertices 18 Symmetry group D3d | |
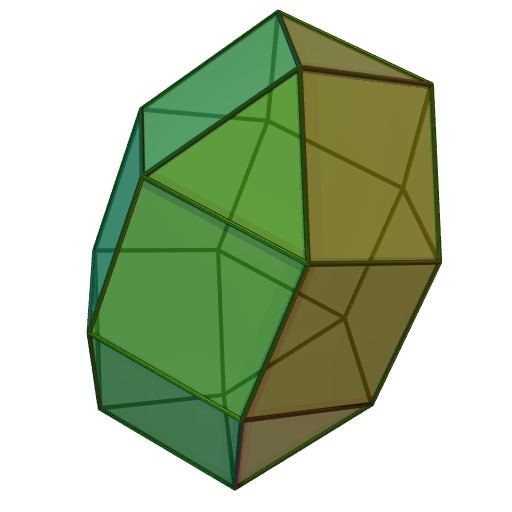 | ||
Type JohnsonJ35 - J36 - J37 Faces 2+6 triangles2.6 squares | ||
In geometry, the elongated triangular gyrobicupola is one of the Johnson solids (J36). As the name suggests, it can be constructed by elongating a "triangular gyrobicupola," or cuboctahedron, by inserting a hexagonal prism between its two halves, which are congruent triangular cupolae (J3). Rotating one of the cupolae through 60 degrees before the elongation yields the triangular orthobicupola (J35).
Contents
A Johnson solid is one of 92 strictly convex polyhedra that have regular faces but are not uniform (that is, they are not Platonic solids, Archimedean solids, prisms or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.
Formulae
The following formulae for volume and surface area can be used if all faces are regular, with edge length a:
Related polyhedra and honeycombs
The elongated triangular gyrobicupola forms space-filling honeycombs with tetrahedra and square pyramids.
