The Douady rabbit is any of various particular filled Julia sets associated with the parameter near the center period 3 buds of Mandelbrot set for complex quadratic map. The rabbit is a parabolic Julia set for internal angle 1/3 (parameter c is a root point between period 1 and period 3 components of Mandelbrot set).
Douady's rabbit or Douady rabbit is named for the French mathematician Adrien Douady.
Fat rabbit or chubby rabbit has c at the root of 1/3-limb of the Mandelbrot set. It has a parabolic fixed point with 3 petals.
There are two common forms for the complex quadratic map
M
. The first, also called the complex logistic map, is written as
z
n
+
1
=
M
z
n
=
γ
z
n
(
1
−
z
n
)
,
where
z
is a complex variable and
γ
is a complex parameter. The second common form is
w
n
+
1
=
M
w
n
=
w
n
2
−
μ
.
Here
w
is a complex variable and
μ
is a complex parameter. The variables
z
and
w
are related by the equation
z
=
−
w
γ
+
1
2
,
and the parameters
γ
and
μ
are related by the equations
μ
=
(
γ
−
1
2
)
2
−
1
4
,
γ
=
1
±
1
+
4
μ
.
Note that
μ
is invariant under the substitution
γ
→
2
−
γ
.
Mandelbrot and filled Julia sets
There are two planes associated with
M
. One of these, the
z
(or
w
) plane, will be called the mapping plane, since
M
sends this plane into itself. The other, the
γ
(or
μ
) plane, will be called the control plane.
The nature of what happens in the mapping plane under repeated application of
M
depends on where
γ
(or
μ
) is in the control plane. The filled Julia set consists of all points in the mapping plane whose images remain bounded under indefinitely repeated applications of
M
. The Mandelbrot set consists of those points in the control plane such that the associated filled Julia set in the mapping plane is connected.
Figure 1 shows the Mandelbrot set when
γ
is the control parameter, and Figure 2 shows the Mandelbrot set when
μ
is the control parameter. Since
z
and
w
are affine transformations of one another (a linear transformation plus a translation), the filled Julia sets look much the same in either the
z
or
w
planes.
The Douady rabbit is most easily described in terms of the Mandelbrot set as shown in Figure 1 (above). In this figure, the Mandelbrot set, at least when viewed from a distance, appears as two back-to-back unit discs with sprouts. Consider the sprouts at the one- and five-o'clock positions on the right disk or the sprouts at the seven- and eleven-o'clock positions on the left disk. When
γ
is within one of these four sprouts, the associated filled Julia set in the mapping plane is a Douady rabbit. For these values of
γ
, it can be shown that
M
has
z
=
0
and one other point as unstable (repelling) fixed points, and
z
=
∞
as an attracting fixed point. Moreover, the map
M
3
has three attracting fixed points. Douady's rabbit consists of the three attracting fixed points
z
1
,
z
2
, and
z
3
and their basins of attraction.
For example, Figure 3 shows Douady's rabbit in the
z
plane when
γ
=
γ
D
=
2.55268
−
0.959456
i
, a point in the five-o'clock sprout of the right disk. For this value of
γ
, the map
M
has the repelling fixed points
z
=
0
and
z
=
.656747
−
.129015
i
. The three attracting fixed points of
M
3
(also called period-three fixed points) have the locations
z
1
=
0.499997032420304
−
(
1.221880225696050
×
10
−
6
)
i
(
r
e
d
)
,
z
2
=
0.638169999974373
−
(
0.239864000011495
)
i
(
g
r
e
e
n
)
,
z
3
=
0.799901291393262
−
(
0.107547238170383
)
i
(
y
e
l
l
o
w
)
.
The red, green, and yellow points lie in the basins
B
(
z
1
)
,
B
(
z
2
)
, and
B
(
z
3
)
of
M
3
, respectively. The white points lie in the basin
B
(
∞
)
of
M
.
The action of
M
on these fixed points is given by the relations
M
z
1
=
z
2
,
M
z
2
=
z
3
,
M
z
3
=
z
1
.
Corresponding to these relations there are the results
M
B
(
z
1
)
=
B
(
z
2
)
o
r
M
r
e
d
⊆
g
r
e
e
n
,
M
B
(
z
2
)
=
B
(
z
3
)
o
r
M
g
r
e
e
n
⊆
y
e
l
l
o
w
,
M
B
(
z
3
)
=
B
(
z
1
)
o
r
M
y
e
l
l
o
w
⊆
r
e
d
.
Note the marvelous fractal structure at the basin boundaries.
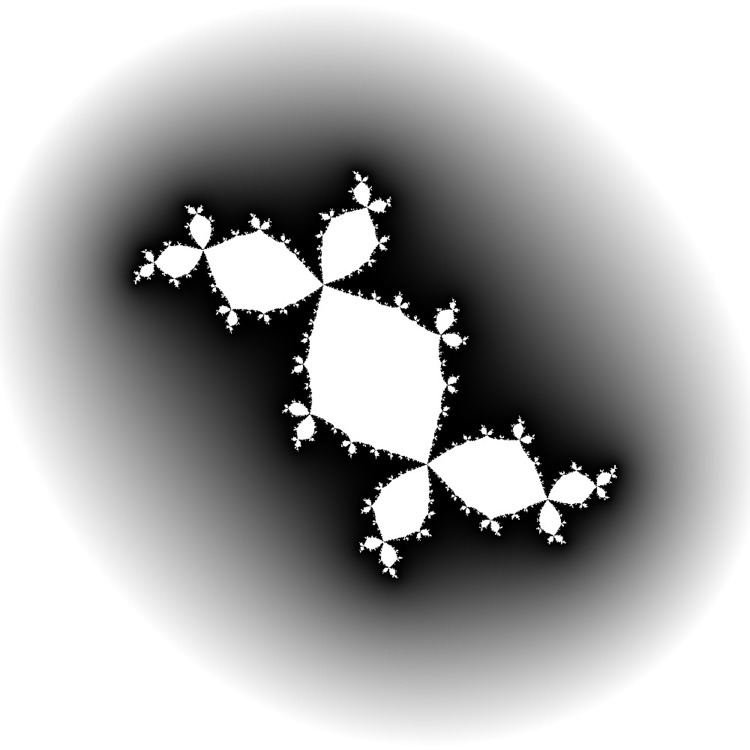
As a second example, Figure 4 shows a Douady rabbit when
γ
=
2
−
γ
D
=
−
.55268
+
.959456
i
, a point in the eleven-o'clock sprout on the left disk. (As noted earlier,
μ
is invariant under this transformation.) The rabbit now sits more symmetrically on the page. The period-three fixed points are located at
z
1
=
0.500003730675024
+
(
6.968273875812428
×
10
−
6
)
i
(
r
e
d
)
,
z
2
=
−
0.138169999969259
+
(
0.239864000061970
)
i
(
g
r
e
e
n
)
,
z
3
=
−
0.238618870661709
−
(
0.264884797354373
)
i
(
y
e
l
l
o
w
)
,
The repelling fixed points of
M
itself are located at
z
=
0
and
z
=
1.450795
+
0.7825835
i
. The three major lobes on the left, which contain the period-three fixed points
z
1
,
z
2
, and
z
3
, meet at the fixed point
z
=
0
, and their counterparts on the right meet at the point
z
=
1
. It can be shown that the effect of
M
on points near the origin consists of a counterclockwise rotation about the origin of
arg
(
γ
)
, or very nearly
120
∘
, followed by scaling (dilation) by a factor of
|
γ
|
=
1.1072538
.