Delannoy number

Example

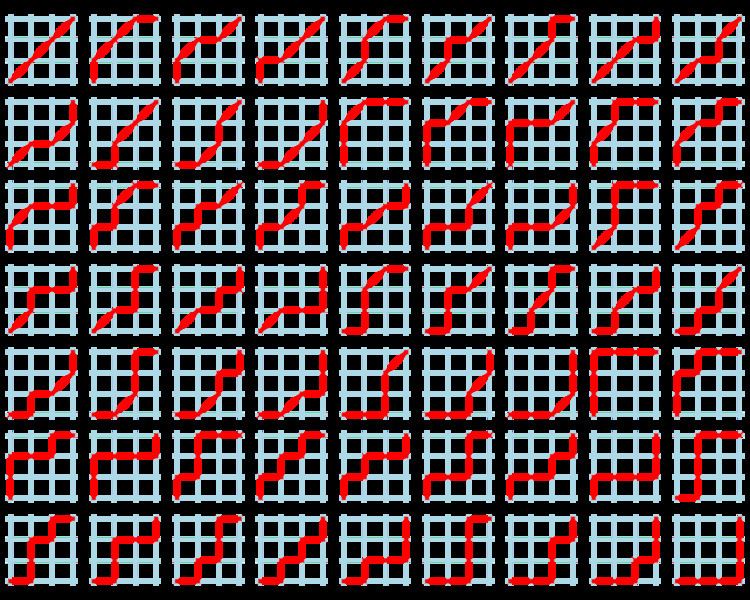
The Delannoy number D(3,3) equals 63. The following figure illustrates the 63 Delannoy paths through a 3 × 3 grid:

The subset of paths that do not rise above the SW–NE diagonal are counted by a related family of numbers, the Schröder numbers.

Delannoy array

The Delannoy array is an infinite matrix of the Delannoy numbers:

In this array, the numbers in the first row are all one, the numbers in the second row are the odd numbers, the numbers in the third row are the centered square numbers, and the numbers in the fourth row are the centered octahedral numbers. Alternatively, the same numbers can be arranged in a triangular array resembling Pascal's triangle, also called the tribonacci triangle, in which each number is the sum of the three numbers above it:

1 1 1 1 3 1 1 5 5 1 1 7 13 7 1 1 9 25 25 9 1 1 11 41 63 41 11 1

Central Delannoy numbers

The central Delannoy numbers D(n) = D(n,n) are the numbers for a square n × n grid. The first few central Delannoy numbers (starting with n=0) are:

1, 3, 13, 63, 321, 1683, 8989, 48639, 265729, ... (sequence A001850 in the OEIS).

Delannoy numbers

For k diagonal (i.e. northeast) steps, there must be m − k steps in the x direction and n − k steps in the y direction in order to reach the point ( m , n ) ; as these steps can be performed in any order, the number of such paths is given by the multinomial coefficient ( m + n − k k , m − k , n − k ) = ( m + n − k m ) ( m k ) . Hence, one gets the closed-form expression

D ( m , n ) = ∑ k = 0 min ( m , n ) ( m + n − k m ) ( m k ) .

An alternative expression is given by

D ( m , n ) = ∑ k = 0 min ( m , n ) ( m k ) ( n k ) 2 k .

And also

D ( m , n ) = ∑ k = 0 n A ( m , k ) ,

where A ( m , k ) is given with (sequence A266213 in the OEIS).

The basic recurrence relation for the Delannoy numbers is easily seen to be

D ( m , n ) = { 1 if  m = 0  or  n = 0 D ( m − 1 , n ) + D ( m − 1 , n − 1 ) + D ( m , n − 1 ) otherwise

This recurrence relation also leads directly to the generating function

∑ m , n = 0 ∞ D ( m , n ) x m y n = ( 1 − x − y − x y ) − 1 .

Central Delannoy numbers

Substituting m = n in the first closed form expression above, replacing k ↔ n − k , and a little algebra, gives

D ( n ) = ∑ k = 0 n ( n k ) ( n + k k ) ,

while the second expression above yields

D ( n ) = ∑ k = 0 n ( n k ) 2 2 k .

The central Delannoy numbers satisfy also a three-term recurrence relationship among themselves,

n D ( n ) = 3 ( 2 n − 1 ) D ( n − 1 ) − ( n − 1 ) D ( n − 2 ) ,

and have a generating function

∑ n = 0 ∞ D ( n ) x n = ( 1 − 6 x + x 2 ) − 1 / 2 .

The leading asymptotic behavior of the central Delannoy numbers is given by

D ( n ) = c α n n ( 1 + O ( n − 1 ) )

where α = 3 + 2 2 ≈ 5.828 and c = ( 4 π ( 3 2 − 4 ) ) − 1 / 2 ≈ 0.5727 .