 | ||
In seven-dimensional geometry, a cantic 7-cube or truncated 7-demicube as a uniform 7-polytope, being a truncation of the 7-demicube.
Contents
A uniform 7-polytope is vertex-transitive and constructed from uniform 6-polytope facets, and can be represented a coxeter diagram with ringed nodes representing active mirrors. A demihypercube is an alternation of a hypercube.
Its 3-dimensional analogue would be a truncated tetrahedron (truncated 3-demicube), and Coxeter diagram or as a cantic cube.
Alternate names
Cartesian coordinates
The Cartesian coordinates for the 1344 vertices of a truncated 7-demicube centered at the origin and edge length 6√2 are coordinate permutations:
(±1,±1,±3,±3,±3,±3,±3)with an odd number of plus signs.
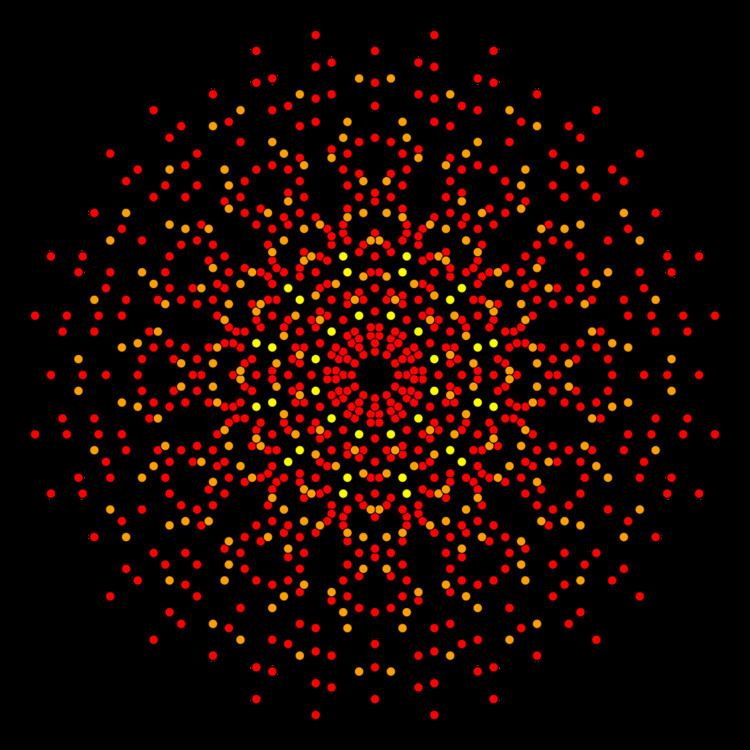
Images
It can be visualized as a 2-dimensional orthogonal projections, for example the a D7 Coxeter plane, containing 12-gonal symmetry. Most visualizations in symmetric projections will contain overlapping vertices, so the colors of the vertices are changed based on how many vertices are at each projective position, here shown with red color for no overlaps.
Related polytopes
There are 95 uniform polytopes with D6 symmetry, 63 are shared by the B6 symmetry, and 32 are unique:
