Schläfli symbol {257} Dual polygon Self | Edges and vertices 257 Internal angle (degrees) ≈178.60° | |
 | ||
Symmetry group Dihedral (D257), order 2×257 | ||
In geometry, a 257-gon (diacosipentacontaheptagon, diacosipentecontaheptagon) is a polygon with 257 sides. The sum of the interior angles of any non-self-intersecting 257-gon is 91800°.
Contents
Regular 257-gon
The area of a regular 257-gon is (with t = edge length)
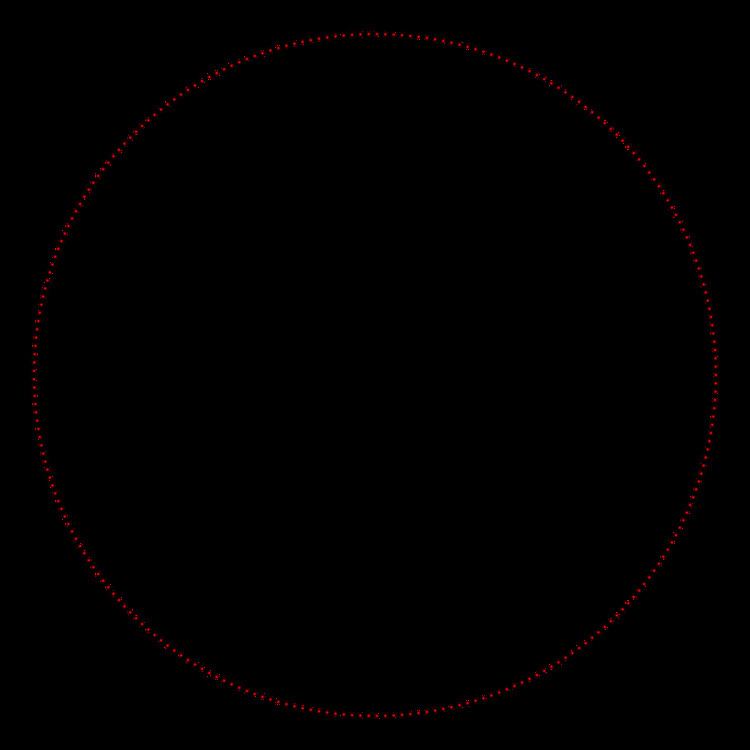
A whole regular 257-gon is not visually discernible from a circle, and its perimeter differs from that of the circumscribed circle by about 24 parts per million.
Construction
The regular 257-gon (one with all sides equal and all angles equal) is of interest for being a constructible polygon: that is, it can be constructed using a compass and an unmarked straightedge. This is because 257 is a Fermat prime, being of the form 22n + 1 (in this case n = 3). Thus, the values
Although it was known to Gauss by 1801 that the regular 257-gon was constructible, the first explicit constructions of a regular 257-gon were given by Magnus Georg Paucker (1822) and Friedrich Julius Richelot (1832). Another method involves the use of 150 circles, 24 being Carlyle circles: this method is pictured below. One of these Carlyle circles solves the quadratic equation x2 + x − 64 = 0.
Symmetry
The regular 257-gon has Dih257 symmetry, order 514. Since 257 is a prime number there is one subgroup with dihedral symmetry: Dih1, and 2 cyclic group symmetries: Z257, and Z1.
257-gram
A 257-gram is a 257-sided star polygon. As 257 is prime, there are 127 regular forms generated by Schläfli symbols {257/n} for all integers 2 ≤ n ≤ 128 as
Below is a view of {257/128}, with 257 nearly radial edges, with its star vertex internal angles 180°/257 (~0.7°).
