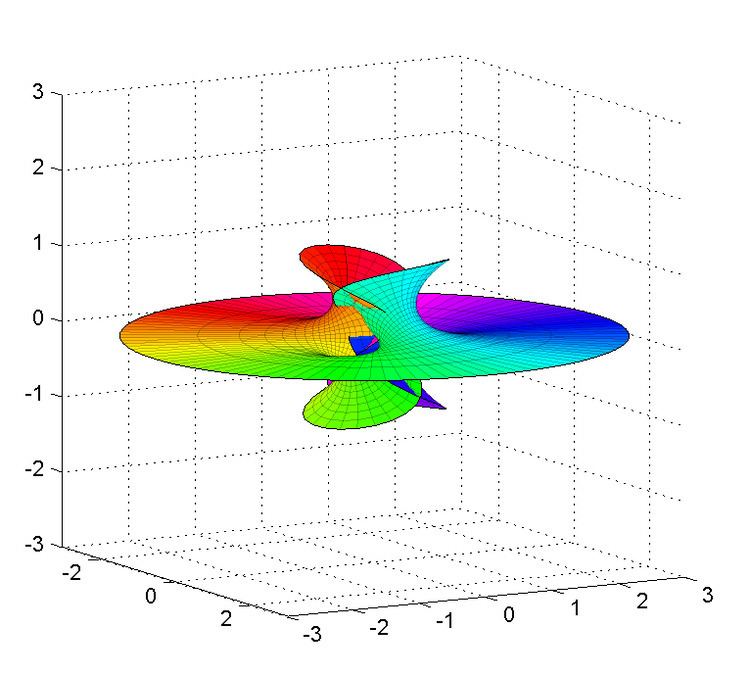
Richmond surface - Alchetron, The Free Social Encyclopedia

In differential geometry, a Richmond surface is a minimal surface first described by Herbert William Richmond in 1904. It is a family of surfaces with one planar end and one Enneper surface-like self-intersecting end.

It has Weierstrass–Enneper parameterization f ( z ) = 1 / z 2 , g ( z ) = z m . This allows a parametrization based on a complex parameter as

X ( z ) = ℜ [ ( − 1 / 2 z ) − z 2 m + 1 / ( 4 m + 2 ) ] Y ( z ) = ℜ [ ( − i / 2 z ) + i z 2 m + 1 / ( 4 m + 2 ) ] Z ( z ) = ℜ [ z m / m ]

The associate family of the surface is just the surface rotated around the z-axis.

Taking m = 2 a real parametric expression becomes:

X ( u , v ) = ( 1 / 3 ) u 3 − u v 2 + u u 2 + v 2 Y ( u , v ) = − u 2 v + ( 1 / 3 ) v 3 − v u 2 + v 2 Z ( u , v ) = 2 u

References

Richmond surface Wikipedia

(Text) CC BY-SA