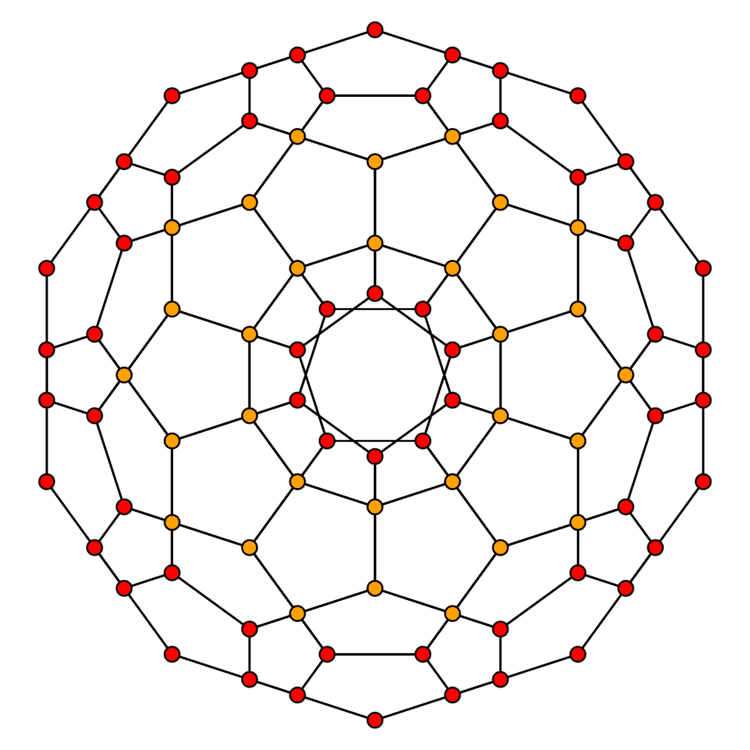
 | ||
In geometry, a rectified 120-cell is a uniform 4-polytope formed as the rectification of the regular 120-cell.
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as tC120.
There are four rectifications of the 120-cell, including the zeroth, the 120-cell itself. The birectified 120-cell is more easily seen as a rectified 600-cell, and the trirectified 120-cell is the same as the dual 600-cell.
Rectified 120-cell
In geometry, the rectified 120-cell or rectified hecatonicosachoron is a convex uniform 4-polytope composed of 600 regular tetrahedra and 120 icosidodecahedra cells. Its vertex figure is a triangular prism, with three icosidodecahedra and two tetrahedra meeting at each vertex.
Alternative names:
References
Rectified 120-cell Wikipedia(Text) CC BY-SA
