Fleiss' kappa

Introduction

Fleiss' kappa is a generalisation of Scott's pi statistic,^[1] a statistical measure of inter-rater reliability.^[2] It is also related to Cohen's kappa statistic and Youden's J statistic which may be more appropriate in certain instances^[3][4]. Whereas Scott's pi and Cohen's kappa work for only two raters, Fleiss' kappa works for any number of raters giving categorical ratings, to a fixed number of items. It can be interpreted as expressing the extent to which the observed amount of agreement among raters exceeds what would be expected if all raters made their ratings completely randomly. It is important to note that whereas Cohen's kappa assumes the same two raters have rated a set of items, Fleiss' kappa specifically allows that although there are a fixed number of raters (e.g., three), different items may be rated by different individuals (Fleiss, 1971, p.378). That is, Item 1 is rated by Raters A, B, and C; but Item 2 could be rated by Raters D, E, and F.

Agreement can be thought of as follows, if a fixed number of people assign numerical ratings to a number of items then the kappa will give a measure for how consistent the ratings are. The kappa, κ , can be defined as,

(1)

The factor 1 − P e ¯ gives the degree of agreement that is attainable above chance, and, P ¯ − P e ¯ gives the degree of agreement actually achieved above chance. If the raters are in complete agreement then κ = 1   . If there is no agreement among the raters (other than what would be expected by chance) then κ ≤ 0 .

An example of the use of Fleiss' kappa may be the following: Consider fourteen psychiatrists are asked to look at ten patients. Each psychiatrist gives one of possibly five diagnoses to each patient. These are compiled into a matrix, and Fleiss' kappa can be computed from this matrix (see example below) to show the degree of agreement between the psychiatrists above the level of agreement expected by chance.

Equations

Let N be the total number of subjects, let n be the number of ratings per subject, and let k be the number of categories into which assignments are made. The subjects are indexed by i = 1, ... N and the categories are indexed by j = 1, ... k. Let n_ij represent the number of raters who assigned the i-th subject to the j-th category.

First calculate p_j, the proportion of all assignments which were to the j-th category:

(2)

Now calculate P i , the extent to which raters agree for the i-th subject (i.e., compute how many rater--rater pairs are in agreement, relative to the number of all possible rater--rater pairs):

(3)

Now compute P ¯ , the mean of the P i 's, and P e ¯ which go into the formula for κ :

(4)

(5)

Worked example

In the following example, fourteen raters ( n ) assign ten "subjects" ( N ) to a total of five categories ( k ). The categories are presented in the columns, while the subjects are presented in the rows. Each cell lists the number of raters who assigned the indicated (row) subject to the indicated (column) category.

Data

See table to the right.

N = 10, n = 14, k = 5

Sum of all cells = 140
Sum of P i = 3.780

Calculations

The value p j is the proportion of all assignments ( N × n , here 10 × 14 = 140 ) that were made to the j th category. For example, taking the first column,

And taking the second row,

In order to calculate P ¯ , we need to know the sum of P i ,

Over the whole sheet,

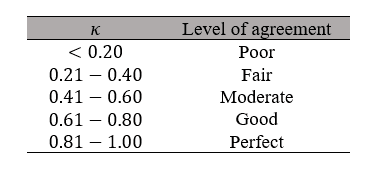
Interpretation

Landis and Koch (1977) gave the following table for interpreting κ values.^[5] This table is however by no means universally accepted. They supplied no evidence to support it, basing it instead on personal opinion. It has been noted that these guidelines may be more harmful than helpful,^[6] as the number of categories and subjects will affect the magnitude of the value. The kappa will be higher when there are fewer categories.^[7]